Tips And Tricks And Shortcuts For Quadratic Equations

Tips and shortcuts to solve the Quadratic Equation questions

• In order to find the sign of roots we use sign given in the equation.

For example: x² + 3x – 4 = 0

The factors are 4 and 1

Now will find the sign of roots. According to the table, if sign given in the equation is + and – then their sign of roots is – and +. Therefore, the roots of the equation are -4 and 1

Type 1: Tricks and Shortcuts for Quadratic Questions

• When one equation has positive roots and other has negative, or equations has same sign roots, then compare the roots and find the relation between them.

Question 1:

Find the correct option given below by solving the following equation  x² – 7x + 10 = 0 and y² + 8y + 15 = 0

Options:

A. x < y B. x > y C. x ≤ y D. x ≥ y E. cannot be determined

Solution:     x² – 7x + 10 = 0…. (1)

Roots of first equation are 5 and 2

Using the table above, we will find the sign of roots. If sign given in the equation is – and + then their sign of roots is + and +

Therefore, the roots of the equation are 5 and 2

Now, y² + 8y + 15 = 0 ……. (2)

Roots of second equation are 3 and 5

If sign given in the equation is + and + then their sign of roots is – and –

Therefore, the roots of the equation are -3 and – 2

Now, here roots are +x1, + x2, – y1, and – y2

This clearly shows that X roots are positive and Y roots are negative. So, x> y

Correct option: B

Type 2: Tips and Tricks To Solve Quadratic Equations Questions

• When roots of the equation are positive and negative and we cannot find the relation between them.

Question 2:

Find the correct option given below by solving the following equation 6x² + 11x – 35 = 0 and  6y² + 5y – 6 = 0

Options:

A. x < y B. x > y C. x ≤ y D. x ≥ y E. cannot be determined

Solution:     6x² + 11x – 35 = 0…. (1)

Roots of first equation are 3.5 and 1.66

Using the table above, we will find the sign of roots. If sign given in the equation is + and – then their sign of roots is – and +

Therefore, the roots of the equation are – 3.5 and 1.66

Now, 6y² + 5y – 6 = 0 ……. (2)

Roots of second equation are 1.5 and 0.66

If sign given in the equation is + and – then their sign of roots is – and +

Therefore, the roots of the equation are – 1.5 and 0.66

Now, here roots are -x₁, + x₂, – y₁, and + y₂

It means x₂> – y₁ and –x₁< + y₂

So, we cannot determine which roots are greater.

Correct option: E

Type 3: Tips and Tricks and Shortcuts for Quadratic Questions

• When we can find a equation where x is a variable and a, b, and c represent constants and D, i.e., Discriminant.

The discriminant is the part of the quadratic formula underneath the square root symbol: b²-4ac. Question 3:

Find the Discriminant and roots of the following equation: 3x² − 5x − 7 = 0.

Solution:

Let us find Discriminant, D = \sqrt{B^{2}-4AC}

D = \sqrt{(-5)^{2}-4(3)(-7)}

D = 109

Therefore, x = \frac{-b \pm \sqrt{}D}{2a}

x = \frac{5 \pm \sqrt{}109}{6}

x = \frac{5 \pm 10.44}{6}

x = \frac{15.44}{6} => x = 2.57 , and

x = \frac{-5.44}{6} => x = – 0.90

So the two roots are 2.57 and -0.90

Some more Questions:

Question 4:

Which method is used to solve quadratic equations that cannot be easily factored or involve complex coefficients?

A) Factoring

B) Quadratic Formula

C) Completing the Square

D) Estimation

Explanation:

The correct answer is option B. The quadratic formula is a universal method used to solve any quadratic equation, regardless of its coefficients or factorability. It provides precise solutions by directly calculating the roots.

Question 5:

For the quadratic equation 3x² + 6x – 9 = 0, what are the roots?

A) x = 1

B) x = -1

C) x = -3

D) x = 3

Explanation:

The correct answer is option C. Divide the entire equation by 3 to simplify it: x² + 2x – 3 = 0. This equation can be factored as (x – 1)(x + 3) = 0. Setting each factor to zero gives x – 1 = 0 and x + 3 = 0. Solving for “x” in both equations yields x = 1 and x = -3. Therefore, the roots are x = -3 and x = 1.

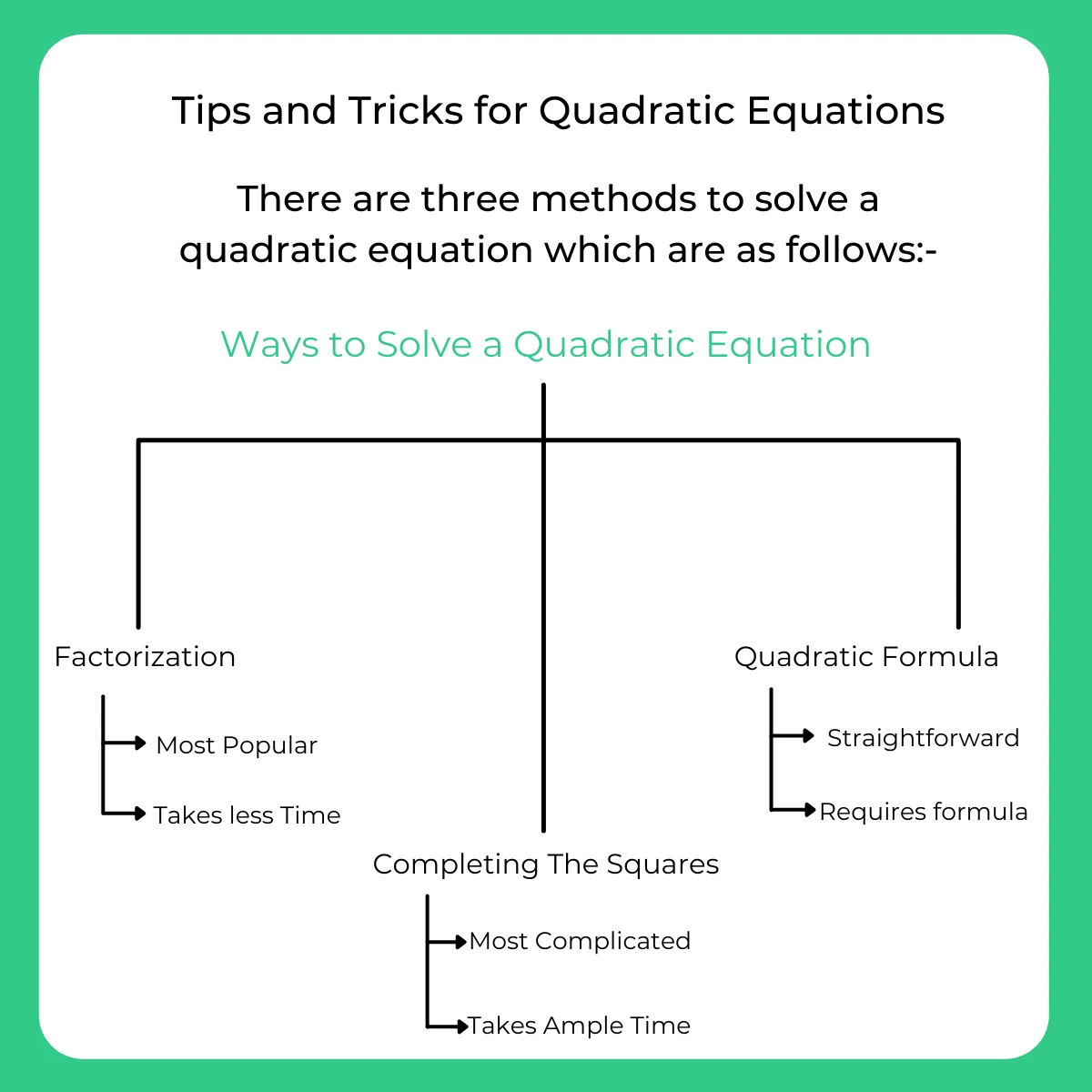
Roadmap for Best Tips: