Formulas For Pipes & Cisterns

December 30, 2021

Concepts of Pipes And Cisterns

As we all know that work and time is considered as one of the major topics of quantitative section for any competitive exam. Similarly, Pipes and Cisterns is major part of work and time. On this page we will see some of the major Formulas For Pipes and Cisterns.

Pipe : There are usually two kinds of Pipes
- Inlet : Inlet pipe is the pipe that fills the cistern or Tank.
- Outlet : Outlet Pipe is the pipe that empties the cistern or tank.
Cisterns : They are large tanks that store rainwater collected from impervious surfaces for domestic uses or for consumption.

Definition of Pipes & Cisterns

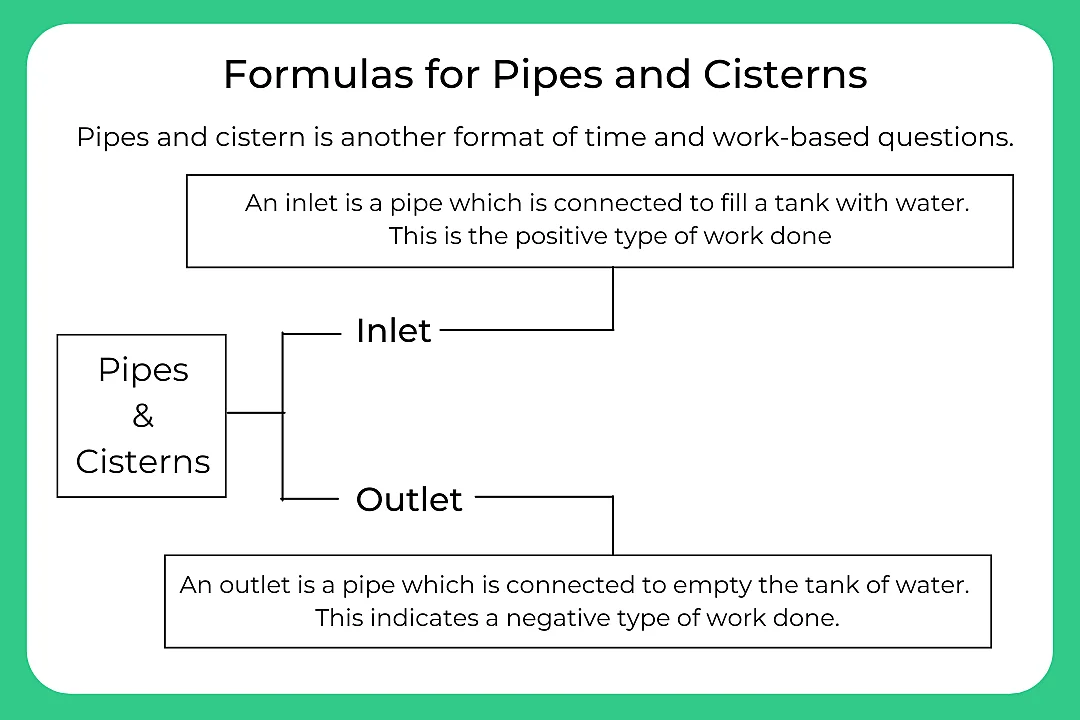
A pipe is connected to a tank or cistern to fill or empty the tank or cistern
- Inlet: A pipe which is connected to fill a tank is known as an inlet.
- Outlet: A pipe which is connected to empty a tank is known as an outlet.
In pipes and cisterns problems – we need to find out what portion of the tank each of the pipes fill or drain in unit time (say in a minute or hour or second) and then perform arithmetic operation on this value.

Formulas for Pipes and Cisterns

If pipe can fill a tank in x hours , then part filled in one hour = $\frac{1}{x}$
If pipe can empty a tank in y hours , then part emptied in one hour = $\frac{1}{y}$
If pipe A can fill a tank in x hours, Pipe B can empty the full tank in y hours (where y > x). Then, on opening both the pipes, the net part filled in one hour= $\frac{1}{x} – \frac{1}{y}$ OR $\frac{xy}{y-x}$ hours
If pipe A can fill a tank in x hours. Pipe B can empty the full tank in y hours (where x > y). Then, on opening both the pipes, the net part filled in one hour= $\frac{1}{y} – \frac{1}{x}$ OR $\frac{yx}{x-y}$ hours.
If pipe A can empty a tank in X hours. Pipe B can empty the same tank in Y hours. Then part of the tank emptied in one hour when both the pipes start working together = $\frac{1}{x} + \frac{1}{y}$

Tips and Tricks and Shortcuts for Pipes and Cisterns

Solve Questions on Pipes and Cisterns