0
Notifications Mark All Read
- Login
- Get Prime
Quants Menu
- HCF and LCM
- Number System
- Number Decimals & Fractions
- Surds and Indices
- Divisibility
- Ages
- LCM
- HCF
- Inverse
- Speed Time and Distance
- Work and Time
- Boats and Streams
- Pipes and Cisterns
- Averages
- Allegations and Mixtures
- Ratio and Proportions
- Simple & Compound Interest
- Simple Interest
- Compound Interest
- Percentages
- Profit & Loss
- Successive Discount 1
- Successive Discount 2
- AP GP HP
- Arithmetic Progressions
- Geometric Progressions
- Harmonic Progressions
- Probability
- Permutation & Combination
- Combination
- Circular Permutation
- Geometry
- Heights and Distances
- Perimeter Area and Volume
- Coordinate Geometry
- Venn Diagrams
- Set Theory
- Algebra
- Linear Equations
- Quadratic Equations
- Logarithms
- Clocks
- Calendars
- Clocks and Calendars
- Finding remainder of large powers
Tips And Tricks And Shortcuts For Combination
How to Solve Inverse Question Quickly in Aptitude
On this page we will be discussing Tips,Tricks and Shorcuts for solving Questions based on Combination. This page will help you to solve maximum number of Question in minimum amount of Time in any Competitive Exam.
Go through this page to get clear understanding of all concepts.
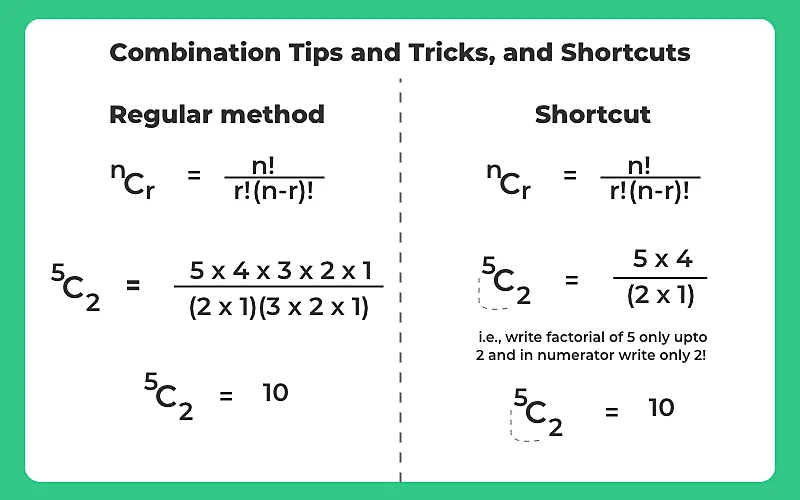
Regular and Shortcut Methods for Combination
Important tips for Combination
- Basically there are two easy tips and tricks on combination
- Repetition of digits is allowed
- Repetition of digits is not allowed
- Here, are quick and easy tips and tricks for you. Combination problems are asked in competitive and recruitment exams.
- In combination, you will learn the combination tricks and tips.
Combination Shortcuts
- Use combinations if a problem calls for the number of ways of selecting objects and the order of selection is not to be counted.
- Summary of formula to use
| Order | Repetition | Formula |
|---|---|---|
| Combination | Yes | ^{r+n-1}C_r |
| Combination | No | ^nC_r |
Combination Tips and Tricks and Shortcuts (with or without repetition)
Prime Course Trailer
Related Banners
Get PrepInsta Prime & get Access to all 200+ courses offered by PrepInsta in One Subscription
Question 1 (with repetition)
Jannat walks in a candy shop. She has enough money to buy 6 candies. The store has 1 pink, 1, green, and 1 white candy. How many different selections can Jannat make?
Solution: r + n – 1Cr = 6 + 3 – 1C6 =8C6
We know that,
^nC_r = \frac{n!}{(n-r)! r! }
8C6= \frac{8!}{(8-6)! 6! } = 28
Question 2 (without repetition)
In how many ways can a swimming coach select 3 swimmers from 8 swimmers?
Solution:
There are eight swimmers out of which three are to be selected
This means 8C3 = \frac{8!}{(8-3)! 3! } = 56
Question 3 (without repetition)
In a group of 10 people, how many different 5-member committees can be formed if it is mandatory to include two specific individuals in each committee?
Solution:
Since two particular people must be included in the committee, we need to select 3 more members to complete the 5-member committee.
The number of ways to select 3 members out of the remaining 10 – 2 = 8 people can be calculated using combinations.
Number of ways to select 3 out of 8 people = ^8C_3=\frac{8!}{3!\times5!}=56
Question 4 (with repetition)
You have four different types of fruits in a basket: apples, oranges, bananas, and grapes. You want to select three fruits from the basket. How many different combinations of three fruits can you make with repetitions allowed?
Solution:
n this case, n = 4 (number of distinct fruits)
r = 3 (number of fruits to be selected).
Using the formula, the number of combinations is:
^{4+3-1}C_3=^6C_3=\frac{6!}{3!\times3!}=20
Question 5 (without repetition)
You have eight friends, and you want to invite three of them to a party. How many different combinations of three friends can you invite?
Solution:
Here, n = 8 (total number of friends) and r = 3 (number of friends to be invited). Applying the combination formula:
^8C_3=\frac{8!}{3!\times(8-3)!}=\frac{8!}{3!\times5!}=56
Get over 200+ course One Subscription
Courses like AI/ML, Cloud Computing, Ethical Hacking, C, C++, Java, Python, DSA (All Languages), Competitive Coding (All Languages), TCS, Infosys, Wipro, Amazon, DBMS, SQL and others
- Probability – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Permutation & Combination – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
Others
- Circular Permutation – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Probability –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Permutation & Combination –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts
Others
- Circular Permutation –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts

 0
0



Login/Signup to comment