How To Solve Linear Equation Questions Quickly

How to Solve Linear Equation Questions & Definition

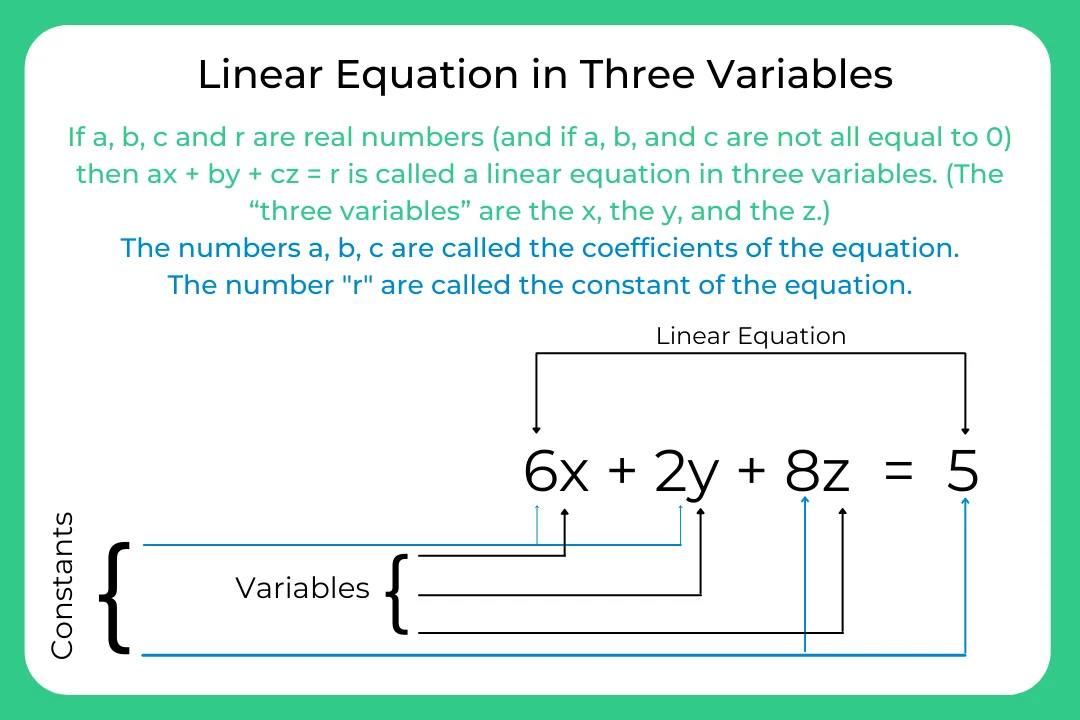
• A linear equation is an equation where variable quantities are in the first power only and whose graph is a straight line.
  
• The above line represent as,  y= mx + c

Methods to solve Linear Equation :

There are mainly 6 methods to solve Linear Equation :

1. Graphical Method
2. Elimination Method
3. Substitution Method
4. Cross Multiplication Method
5. Matrix Method
6. Determinants Method

Let’s explain each method in terms of solving linear equations:

1. Graphical Method: The graphical method involves graphing both sides of a linear equation on the same coordinate plane and finding the point(s) where the graphs intersect. The solution to the equation is the x-coordinate (and corresponding y-coordinate) of the point(s) of intersection. In this method, the graphical representation of the equation visually illustrates the solution(s) to the equation.

2. Elimination Method: In the elimination method, you aim to eliminate one variable by adding or subtracting multiple equations to make one variable’s coefficient cancel out. This process leads to an equation with only one variable, which can be easily solved. After finding the value of one variable, you can substitute it back into one of the original equations to find the value of the other variable.

3. Substitution Method: The substitution method involves solving one variable in terms of the other from one of the equations and then substituting this expression into the other equation. By doing so, you reduce the system of equations to a single equation with one variable, which can then be solved easily.

4. Cross Multiplication Method: The cross multiplication method is used to solve equations with fractions. When you have an equation with fractions, you cross-multiply by multiplying the numerator of one fraction with the denominator of the other and vice versa. This process eliminates the fractions and simplifies the equation, making it easier to solve.

5. Matrix Method: In the matrix method, you represent a system of linear equations using matrices and use matrix operations to solve for the variables. This method is particularly useful when dealing with large systems of equations, as it simplifies the process of solving using matrices and their properties.

6. Determinants Method: The determinants method involves using the concept of determinants from linear algebra to solve a system of linear equations. You form a matrix with the coefficients of the variables, a matrix with the constants on the right-hand side, and then find the determinants of these matrices. By using Cramer’s rule or other determinant-based methods, you can solve for the variables.

Type 1: Solve Linear Equations Questions Quickly, Find the value of x or y.

Question 1. If 3a + 7b = 75 and 5a – 5b = 25, what is the value of a + b? 

Options:

A. 11

B. 6

C. 5

D. 17

Solution:    3a + 7b = 75 ……(1)

5a – 5b = 25 (divide the equation by 5)

we get, a – b = 5 …….(2)

Now multiplying eq. (2) by 7

and add to eq. (1), we get

3a + 7b = 75

7a – 7b = 35

On solving

10a = 110

a = \frac{110}{10}

a = 11

Now put the value of a in eq (2)

11 – b = 5

b = 11 – 5

b = 6

Therefore, a = 11 and b = 6

The value of a + b = 6 + 11 = 17

Correct option: D

Question 2. If 2^{x + y} = 16 and 16^{x – y} = 2, then find the value of x?

Options:

A. \frac{1}{4}

B. \frac{17}{4}

C. \frac{17}{8}

D. 4

Solution:     Given, 2^{x + y} = 16

2^{x + y} = 2⁴

x + y = 4….(1)

Now, 16^{x – y} = 2

(2⁴) ^{x – y} = 2¹

x – y = \frac{1}{4} ….(2)

On solving equation 1 and 2

We get,

2x = \frac{17}{4}

x = \frac{17}{4 × 2} = \frac{17}{8}

Correct option: C

Question 3. The system of equations 3a + 5b = 6 and 6a + 10y = 6 has

Options:

A. No solution

B. One solution

C. Two solution

D. Infinite solution

Solution:     \frac{a_{1}}{a_{2}} = 3/6 = 1/2

 \frac{b_{1}}{b_{2}}= 5/10 = 1/2

 \frac{c_{1}}{c_{2}} =  \frac{6}{6}= 1

 \frac{a_{1}}{a_{2}} =  \frac{b_{1}}{b_{2}} ≠  \frac{c_{1}}{c_{2}}

Therefore, there is no solution

Correct option: A