- Home
- Allegations and Mixtures
- AP GP HP
- Arithmetic Progressions
- Averages
- Boats and Streams
- Geometric Progressions
- Harmonic Progressions
- Clocks
- Calendar
- Clocks and Calendars
- Compound Interest
- Simple Interest
- Simple Interest and Compound Interest
- Linear Equations
- Quadratic Equations
- Co-ordinate geometry
- Perimeter Area Volume
- Divisibility
- HCF and LCM
- HCF
- LCM
- Number System
- Percentages
- Permutations Combinations
- Combinations
- Piipes and Cisterns
- Probability
- Work and Time
- Succesive Discounts
- Heights and Distance
- Decimals and Fractions
- Logarithm
- Venn Diagrams
- Geometry
- Set Theory
- Problem on Ages
- Inverse
- Surds and Indices
- Profit and Loss
- Speed, Time and Distance
- Algebra
- Ratio & Proportion
- Number, Decimals and Fractions
Programming
Aptitude
Syllabus
Interview Preparation
Interview Exp.
Off Campus
Formulas For Algebra
Basic Concepts and Formulas For Algebra
Definition of Algebra: –
Algebra is the study of mathematical symbols. It also comprises of the rules that are used to manipulate these symbols. This page is all about use and formulas For Algebra.
The study comprises of almost everything from elementary equation solving to the study of abstractions such as groups, rings, and fields.
Use and Formulas For Algebra :-
Algebra is an important life skill worth understanding well. It moves us beyond basic math and prepares us for statistics and calculus. It is useful for many jobs some of which a student may enter as a second career.
Algebra is useful around the house and in analyzing information in the news.
Algebraic Expressions:-
Here we have listed some of the most important Formulas For Algebra:-
- a2 – b2 = (a – b)(a + b)
- (a+b)2 = a2 + 2ab + b2
- a2 + b2 = (a – b)2 + 2ab
- (a – b)2 = a2 – 2ab + b2
- (a + b + c)2 = a2 + b2 + c2 + 2ab + 2ac + 2bc
- (a – b – c)2 = a2 + b2 + c2 – 2ab – 2ac + 2bc
- (a + b)3 = a3 + 3a2b + 3ab2 + b3 , which can be also denoted as – (a + b)3 = a3 + b3 + 3ab(a + b)
- (a – b)3 = a3 – 3a2b + 3ab2 – b3
- a3 – b3 = (a – b)(a2 + ab + b2)
- a3 + b3 = (a + b)(a2 – ab + b2)
- (a + b)4 = a4 + 4a3b + 6a2b2 + 4ab3 + b4)
- (a – b)4 = a4 – 4a3b + 6a2b2 – 4ab3 + b4)
- a4 – b4 = (a – b)(a + b)(a2 + b2)
- a5 – b5 = (a – b)(a4 + a3b + a2b2 + ab3 + b4)
Properties and Formulas For Algebra
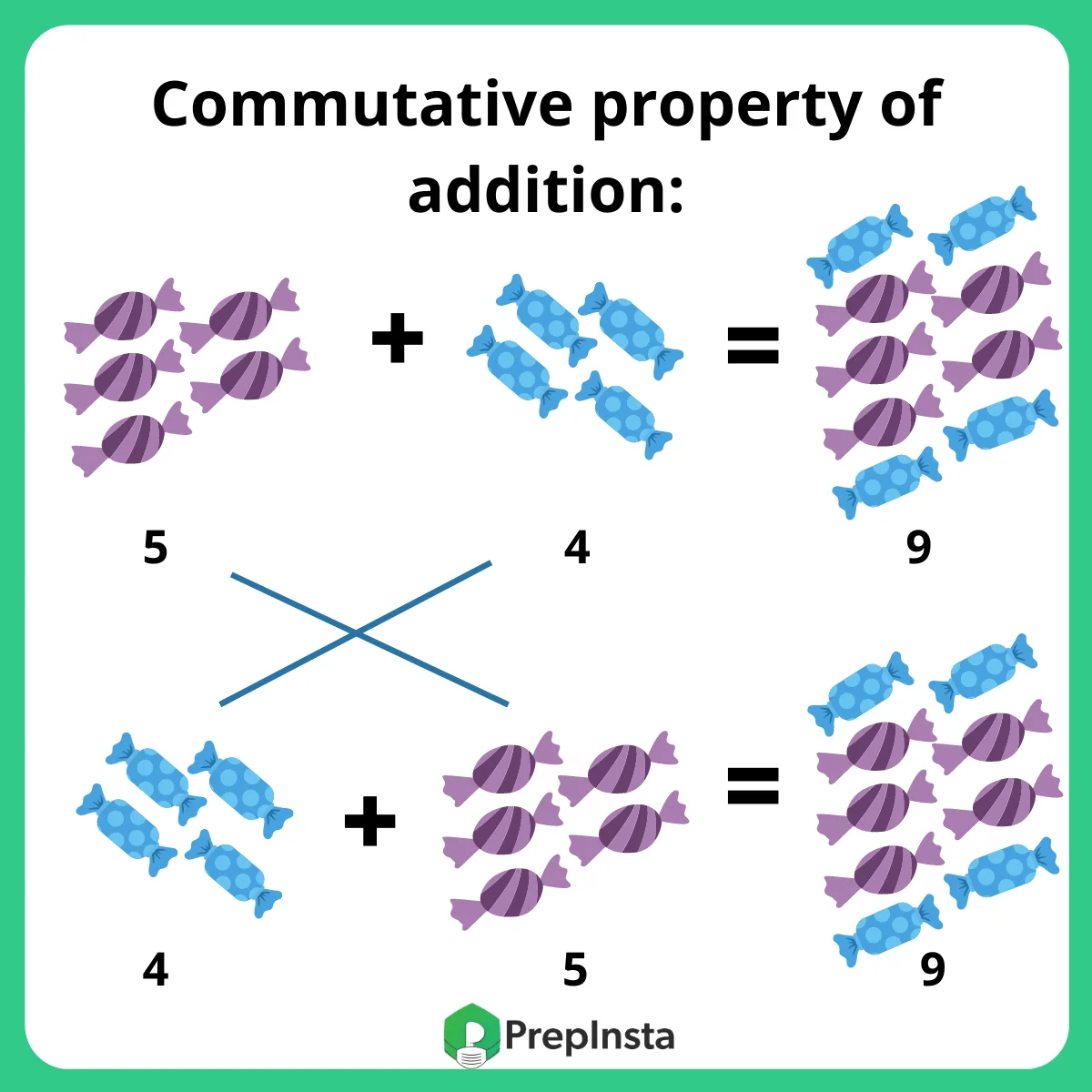
1.Commutative property of addition:
a + b = b + a
The sum of the expression does not changes if the order of the elements are changed. The elements can be expressions or numbers.
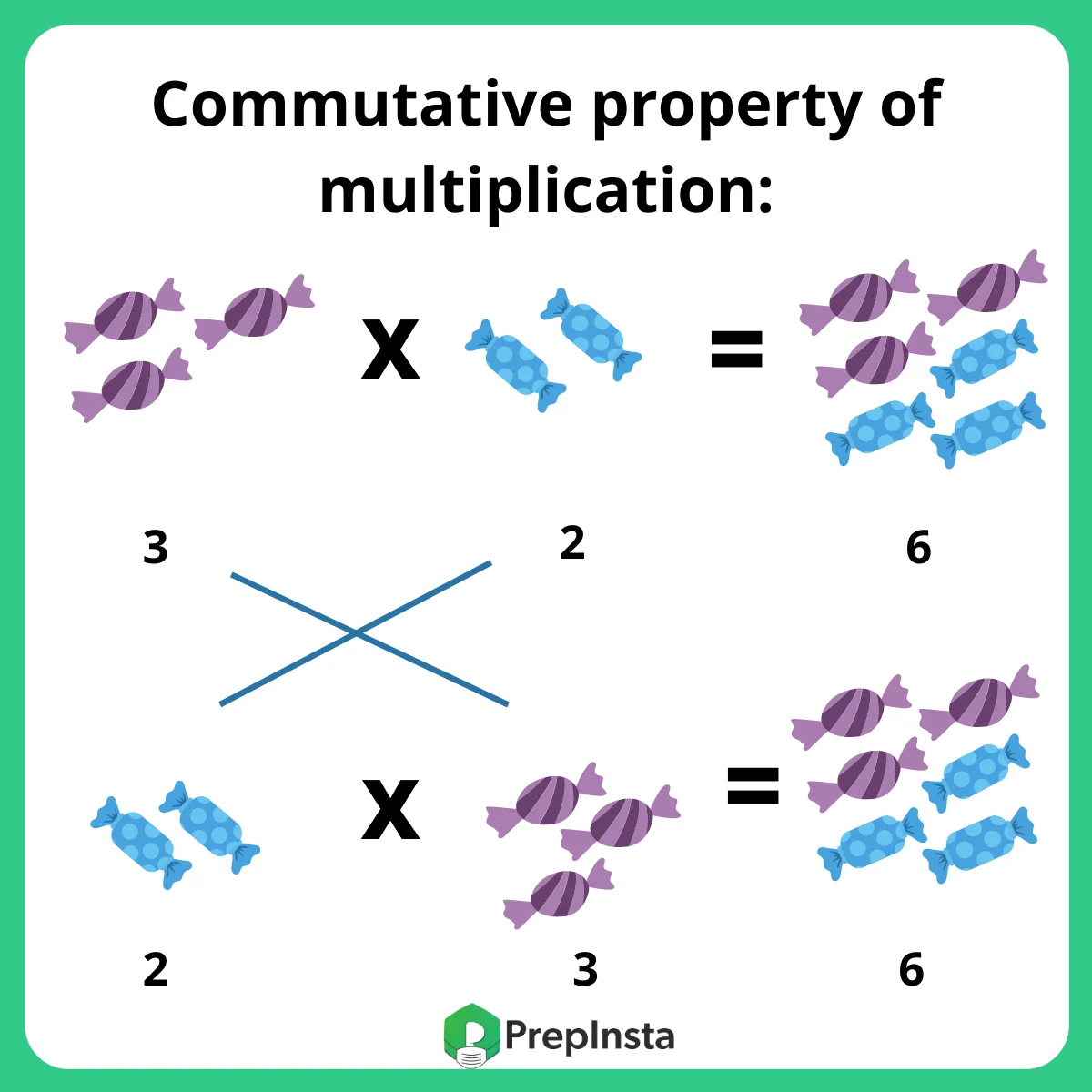
2. Commutative Property of Multiplication:
a x b = b x a
When the order of the factors are changed, the product does not change. These factors can be numbers or expressions
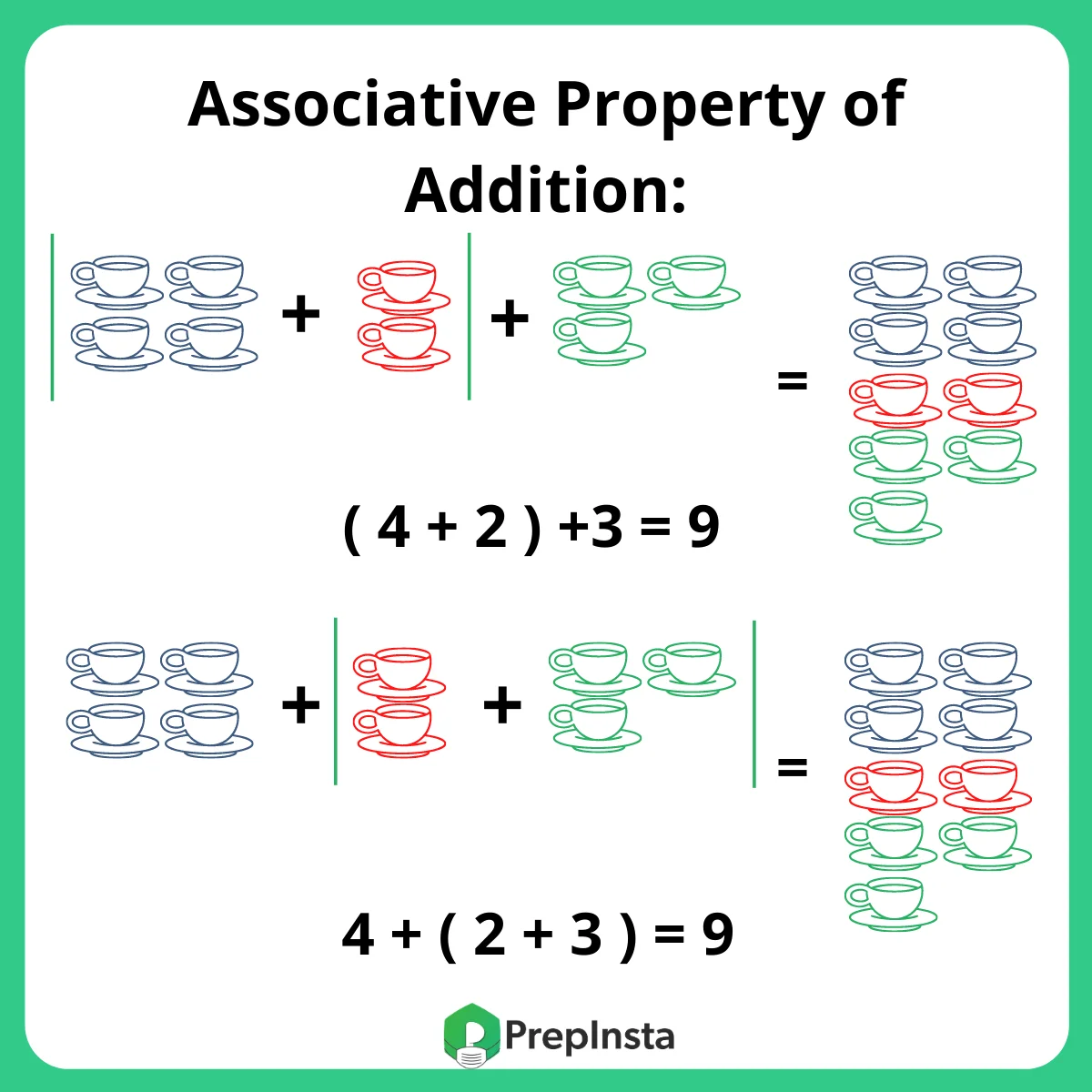
3. Associative Property of Addition:
(a + b)+ c = a + (b + c)
The property defines that when two or more numbers are grouped together which are performing basic arithmetic addition, their order does not play a significant role in the result.
4. Associative property of Multiplication:
(a x b) xc = a x (b x c)
Associative property states that when two or more elements are grouped together in the basic arithmetical multiplication, their order does not change the final result. Also, in this case, the grouping is usually done by parenthesis.
5. Distributive properties of Addition and Multiplication:
a × (b + c) = a × b + a × c
and
(a + b) × c = a × c + b × c
The distributive property says that the product of a single term and a sum or a difference of two or more terms present in the bracket is same as multiplying each of the element by a single term and them adding and subtracting the products.
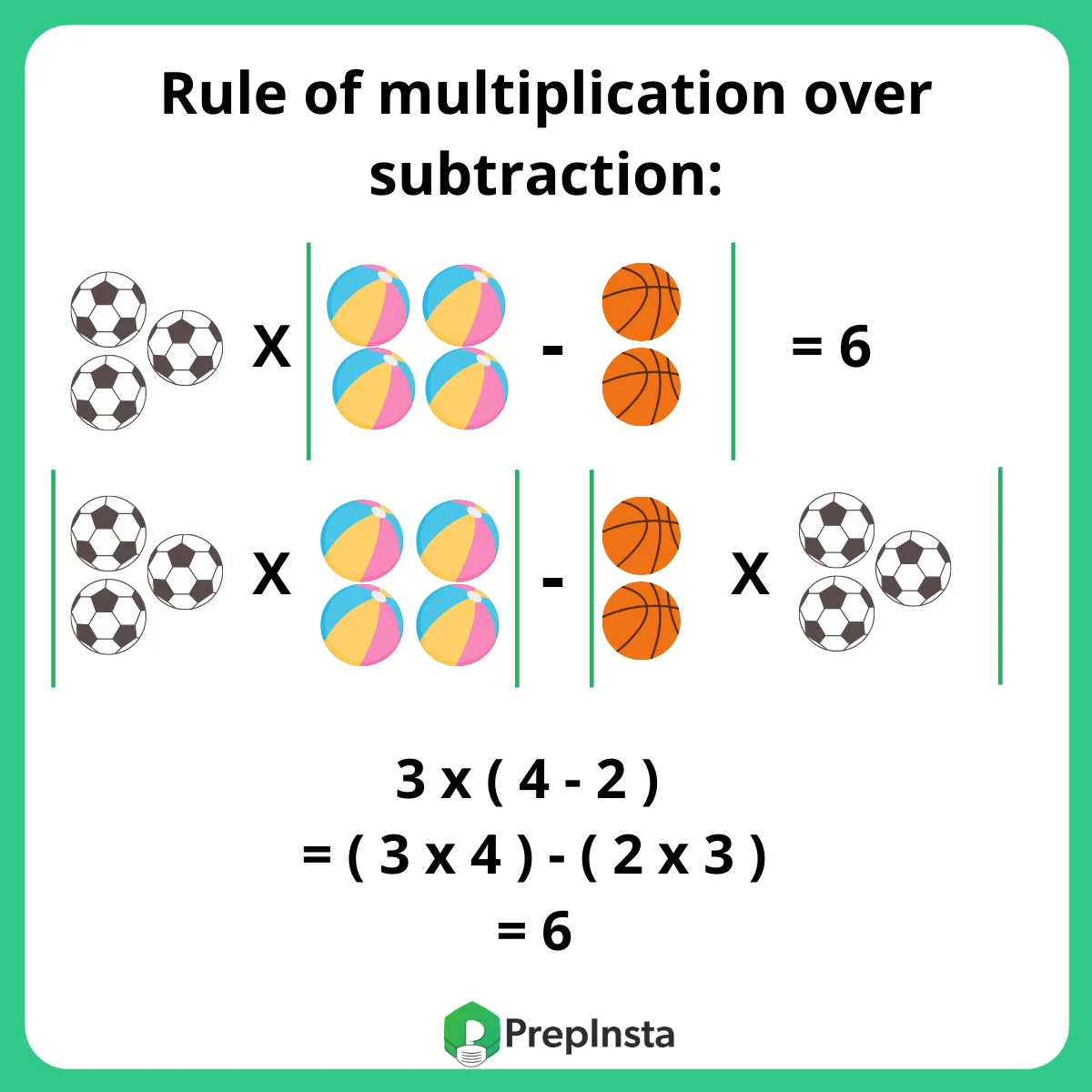
6. Rule of multiplication over subtraction:
If p, q, and r are any numbers, then, p (q-r) = p*q – p*r. Similarly, in the addition rule, the distribution for multiplication over subtraction can be done in left distribution and right distribution.
If p* (q-r) = (p * q) – (p*r)- Left distributive law
and
If (p-q)*r = (p*r) – (q*r)- Right distributive law
Login/Signup to comment