Quants Menu
- HCF and LCM
- Number System
- Number Decimals & Fractions
- Surds and Indices
- Divisibility
- Ages
- LCM
- HCF
- Inverse
- Speed Time and Distance
- Work and Time
- Boats and Streams
- Pipes and Cisterns
- Averages
- Allegations and Mixtures
- Ratio and Proportions
- Simple & Compound Interest
- Simple Interest
- Compound Interest
- Percentages
- Profit & Loss
- Successive Discount 1
- Successive Discount 2
- AP GP HP
- Arithmetic Progressions
- Geometric Progressions
- Harmonic Progressions
- Probability
- Permutation & Combination
- Combination
- Circular Permutation
- Geometry
- Heights and Distances
- Perimeter Area and Volume
- Coordinate Geometry
- Venn Diagrams
- Set Theory
- Algebra
- Linear Equations
- Quadratic Equations
- Logarithms
- Clocks
- Calendars
- Clocks and Calendars
- Finding remainder of large powers
PREPINSTA PRIME
Formulas For Inverse
Formulas for Inverse in Aptitude
Here , In this Page Formulas for Inverse is given. Inverse is a Function that Reverses another Function.
If the function is denoted by f(x) then inverse is denoted by f^{-1}(x)
Inverse functions gives lots of troubles so here’s a swift run down of what an inverse function is, and how to find it.
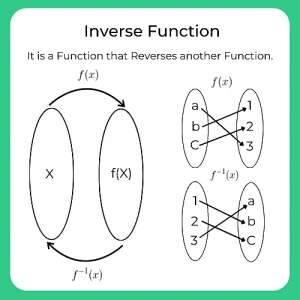
Types of Inverse function
| Function | Inverse Function | Note |
|---|---|---|
| + | – | |
| * | / | Don’t divide by 0 |
| 1/x | 1/y | x and y not equal to 0 |
| x^{2} | \sqrt{y} | x and y ≥ 0 |
| x^{n} | y^{1/n} | n is not equal to 0 |
| e^{x} | In(y) | y > 0 |
| a^{x} | log a(y) | y and a > 0 |
| Sin x | Sin^{-1}(y) | – π/2 to + π/2 |
| Cos x | Cos^{-1}(y) | 0 to π |
| tan x | tan^{-1}(y) | – π/2 to + π/2 |
Inverse Formulas Example-
f(x) = 3x -2 and g(x) =\frac{x}{3} + \frac{2}{3}
(f∘g)(x)= (g∘f)(x) = x
- The first case is really,
(g∘f)(−1)=g[f(−1)]=g[−5]=−1
- The second case is really,
(f∘g)(2) = f(2) = f[g(2)] = f [ \frac{4}{3} \ ] = 2
- A function is called one-to-one if no two values of x produce the same y. Mathematically this is the same as saying
f(x1)\neq f(x2) whenever x1 \neq x2 - Inverse functions are one to one functions f(x) and g(x) if
(f∘g)(x) = x and (g∘f)(x) = x, then we can say f(x) and g(x) are inverse to each other. - g(x) is inverse of f(x) and denoted by
g(x)= f-1 (x) - Like wise f(x) is the inverse of g(x) are denoted by
f(x)= g-1 (x)
For the two functions that we started off this section with we could write either of the following two sets of notation.
f(x) = 3x -2
f-1 (x) = \frac{x}{3} + \frac{2}{3}
g(x) = \frac{x}{3} + \frac{2}{3}
g-1 (x) = 3x-2
Inverse Formulas with solved Examples
Given f(x) 3x – 2 find \mathbf{f^{-1} (x)}
Solution:
we’ll first replace f(x) with y
y= 3x-2
Next, replace x with y and all y with x.
x = 3y -2
x + 2 = 3y
\frac{1}{3} (x+2) = y
\frac{x}{3} + \frac{2}{3} = y
Finally, replace y with f^{-1} (x)
f^{-1} (x) = \frac{x}{3} \ + \frac{2}{3}
f∘f^{-1} (x) = f[f^{-1}(x)]
= f[ \frac{x}{3} \ + \frac{2}{3} \ ]
= 3 ( \frac{x}{3} \ + \frac{2}{3} \ ) – 2
= x + 2 – 2
= x
Question and Answers for Inverse
Question 1 : Let f(x) = x^3 + 2x + 1. Find the domain of the inverse function of f(x).
A. (-\infty, \infty)
B. [-1, \infty)
C. (-\infty, -1] U [0, \infty)
D. (-\infty, -1) U [0, \infty)
Explanation:
To find the domain of the inverse function of f(x), we first need to find the inverse function of f(x) and then find the domain of the inverse function.
Since finding the inverse function is a bit complicated for a cubic function, we can use a shortcut by noticing that f(x) is an increasing function on its domain (which is (-\infty, \infty)), which means that its inverse function also has a domain of (-\infty, \infty). Therefore, the answer is (a).
Question 2 : Let f(x) = (2x – 1) / (3x + 4). Find inverse of f(x)
A. f^{-1}(x) = \frac{1+4x}{2-3x}
B. f^{-1}(x) = \frac{2-3x}{1+4x}
C. f^{-1}(x) = \frac{4x+1}{3x-2}
D. f^{-1}(x) = \frac{3x+4}{2x-1}
Solution:
To find f^{-1}(x), we switch x and y and solve for y:<br /> x =\frac{2y – 1}{3y + 4} ,
x(3y + 4) = 2y – 1,
3xy + 4x = 2y – 1,
y(2 – 3x) = 4x + 1,
y =\frac{4x + 1}{2 – 3x} ,
Therefore, f^{-1}(x) = (4x + 1) / (2 – 3x)
Question 3 : Let f(x) = 3x – 1. Find the inverse function of f(x).
A. f^{-1}(x) = (x + 1)/3
B. f^{-1}(x) = (x – 1)/3
C. f^{-1}(x) = (x – 1)*3
D. f^{-1}(x) = (x + 1)*3
Explanation:
To find the inverse function of f(x),
we need to solve for x in terms of f(x) and then interchange x and f(x).
So, we start with y = 3x – 1, then solve for x as x = (y + 1)/3.
Next, we replace x with f^{-1}(x) and y with x in the equation to get
f^{-1}(x) = (x + 1)/3
Question 4 : Let f(x) = 3x + 2. Find inverse of this function
A. (x – 2) / 3
B. (x + 2) / 3
C. (x – 3) / 2
D. (x + 3) / 2
Solution:
To find the inverse of f(x), we switch x and y and solve for y:
x = 3y + 2,
x – 2 = 3y,
y = (x – 2) / 3.
Therefore, Inverse of f(x) = (x – 2) / 3.
Question 5 : Let f(x) = 2x – 5. Find f^{-1}(x).
A. f^{-1}(x) = \frac{x}{2} – 5
B. f^{-1}(x) = \frac{x+5}{2}
C. f^{-1}(x) = \frac{2}{x} – 5
D. f^{-1}(x) = \frac{x-5}{2}
Solution:
To find f^{-1}(x), we switch x and y and solve for y:
x = 2y – 5,
x + 5 = 2y,
y = (x + 5) / 2.
Therefore, f^{-1}(x) = \frac{x+5}{2}
Prime Course Trailer
Related Banners
Get PrepInsta Prime & get Access to all 200+ courses offered by PrepInsta in One Subscription
Get over 200+ course One Subscription
Courses like AI/ML, Cloud Computing, Ethical Hacking, C, C++, Java, Python, DSA (All Languages), Competitive Coding (All Languages), TCS, Infosys, Wipro, Amazon, DBMS, SQL and others
- HCF and LCM – Questions Formulas | How to Solve Quickly | Tricks & Shortcuts
- Number System – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Number Decimals & Fractions – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Surds and Indices – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Divisibility – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Ages – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- LCM – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- HCF – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- HCF and LCM –
Questions
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Number System –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Number Decimals & Fractions –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Surds and Indices-
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Divisibility –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Ages –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts

 Apply For Jobs
Apply For Jobs Get Hiring Updates
Get Hiring Updates




Login/Signup to comment