Quants Menu
- HCF and LCM
- Number System
- Number Decimals & Fractions
- Surds and Indices
- Divisibility
- Ages
- LCM
- HCF
- Inverse
- Speed Time and Distance
- Work and Time
- Boats and Streams
- Pipes and Cisterns
- Averages
- Allegations and Mixtures
- Ratio and Proportions
- Simple & Compound Interest
- Simple Interest
- Compound Interest
- Percentages
- Profit & Loss
- Successive Discount 1
- Successive Discount 2
- AP GP HP
- Arithmetic Progressions
- Geometric Progressions
- Harmonic Progressions
- Probability
- Permutation & Combination
- Combination
- Circular Permutation
- Geometry
- Heights and Distances
- Perimeter Area and Volume
- Coordinate Geometry
- Venn Diagrams
- Set Theory
- Algebra
- Linear Equations
- Quadratic Equations
- Logarithms
- Clocks
- Calendars
- Clocks and Calendars
- Finding remainder of large powers
PREPINSTA PRIME
Formulas for Simple Interest Problems
Formulas to Solve Simple interest Problems in Aptitude
Simple interest is a easy method of calculating the interest charge on a loan. First, it is important to recall the concept of interest and ways to calculate it. This Page from here on contains Formulas and definition of Simple Interest.

Simple Interest Formulas
- Simple Interest is the rate at which we lend or borrow money. In simple terms, when a lender lends money to a borrower, the borrower has to pay an extra amount of money to the lender. This extra amount of money is called interest. The interest on a sum borrowed for a certain period is called simple interest.
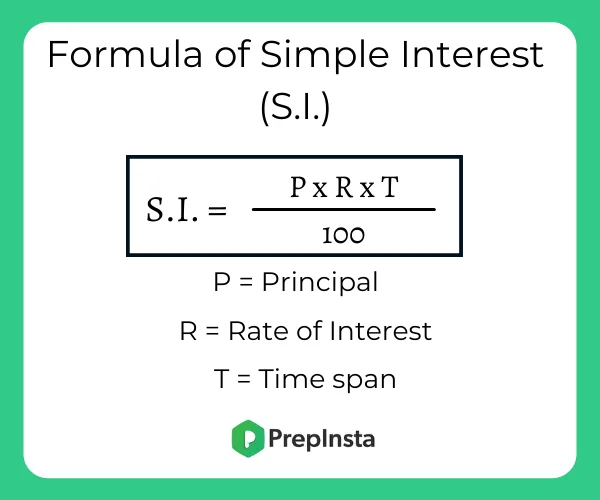
- After the calculation for S.I. is done, the principal has to be added to it to get the total amount that the borrower has to give or the lender will collect. This is called total amount and its formula is given as:
Notations in S.I. Formula:
| S.I | Simple Interest |
| P | Principal Amount |
| A | Total Amount |
| R | Rate of Interest |
| T | Time (in Years) |
Using the above notations, the formula for S.I. becomes,
S.I.=\frac{P * R * T}{100}
This formula can be used to find the missing parameters while calculating the interest or total amount. Thus, the reduced forms of this formula are:
To calculate the Interest, the formula becomes:
- I = PTR/100
To calculate the Principal Amount, the formula is:
- P = (I × 100) / RT
To find the rate of interest, the formula will be:
- R = (I × 100)/ PT
- R (in decimal ) = I/PT
Thus, the rate of interest in percent is given by:
- R = R * 100
To get the time, formula is:
- T = (I × 100) / PR
Prime Course Trailer
Related Banners
Get PrepInsta Prime & get Access to all 200+ courses offered by PrepInsta in One Subscription
Formulas based Questions on Simple Interest
Example 1. Find the simple interest on Rs. 65,000 at 6(2/3)% per annum for a period of 9 months?
Options
(A) 3520
(B) 3250
(C) 2350
(D) 5320
Solution Principle = 65,000
Rate = 6 \frac{2}{3} \
Time = 9 months
Principle = 65,000
Rate = \frac{20}{3} \
Time = \frac{3}{4} \
S.I = \frac{P*R*T}{100} \
= Rs. [65000×(20/3)×(3/4)×(1/100)]
= 3250
Correct Option(B)
Example 2. What sum of money will amount to Rs. 520 in 5 years and Rs. 568 in 7 years at simple interest?
Options
(A) 350
(B) 400
(C) 550
(D) 500
Solution Amount in 5 years = Rs 520
Amount in 7 years = Rs 568
2 years S.I = 568-520 = 48
Simple Interest for 1 years = \frac{48}{2} \ = 24
5 years amount = Rs 520
For 1 years = 5*24 = 120
P = A – S.I = 520-120
P = 400
Correct Option(B)
Example 3 : At what rate, percent per annum will a sum of money triple in 13 years approximately.
Options
(A) 2.25%
(B) 8.98%
(C) 15.38%
(D) None
Solution : Let principal = P, Then, S.I.= 2P and Time = 13 years
We know that S.I. = \frac{PTR}{100}
Rate = \frac{100*2P}{P*13}% = 15.38% per annum.
Correct option : 15.38%
Example 4 : A sum was put at simple interest at a certain rate for 9 years. Had it been put at a 5% higher rate, it would have fetched Rs 540 more. Find the sum.
Options
(A) 5278 rs
(B) 1200 rs
(C) 627 rs
(D) 3200 rs
Solution: Let sum = P and original rate = R. Then
[\frac{(P \times (R+5) \times 9)}{100}] – [\frac{(P \times R \times 9)}{100}] = 540
9P \times (R+5) – 9PR = 54000
9PR + 45P – 9PR = 54000
45P = 54000
P = 1200
Correct Option : 3200 Rs
Example 5 : A sum of money at simple interest amounts to Rs. 873 in 6 years and to Rs. 923 in 9 years. The sum is:
Options
(A) 624 rs
(B) 573 rs
(C) 362 rs
(D) none
Solution: S.I. for 1 year = Rs. (923 – 873) = Rs. 50.
S.I. for 6 years = Rs.(50 * 6) = Rs. 300.
Principal = Rs. (873 – 300) = Rs. 573
Correct Option: 573 Rs
Also Check Out
Get over 200+ course One Subscription
Courses like AI/ML, Cloud Computing, Ethical Hacking, C, C++, Java, Python, DSA (All Languages), Competitive Coding (All Languages), TCS, Infosys, Wipro, Amazon, DBMS, SQL and others
- Averages – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Allegations and Mixtures – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Ratio and Proportions – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Simple & Compound Interest – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Compound Interest – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Percentages – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Profit & Loss – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
Others
- Allegations and Mixtures – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Ratio and Proportions – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Simple & Compound Interest – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Averages – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Compound Interest – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Percentages – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Profit & Loss – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts
Others

 Apply For Jobs
Apply For Jobs Get Hiring Updates
Get Hiring Updates




Login/Signup to comment