Height And Distance Formulas

March 7, 2024

Height and Distances related Formulas

Trigonometry was invented because its need arose in astronomy. Since then the astronomers have used it, for instance, to calculate distances from the Earth to the planets and stars. In this page we are going to learn about various Formulas for Height and Distances which will include different types of trigonometric ratios and figures. For your knowledge 

formulas for heights and distance

Formulas of Height and Distance

Height and Distance Formulas

  • There are basically two terms associated with heights and distances which are as follows :
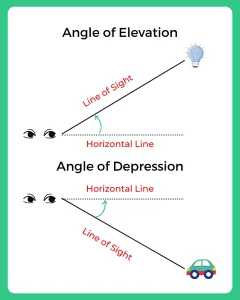
    • Angle of Elevation.
    • Angle of Depression.

Formulas for Angle of Elevation

  • The Angle of elevation of the point viewed is the angle formed by the line of sight with the horizontal when the point being viewed is above the horizontal level, i.e., the case when we raise our head to look at the object

Formulas for Angle of Depression

  • The angle of depression of a point on the object being viewed is the angle formed by the line of sight with the horizontal when the point is below the horizontal level, i.e., the case when we lower our head to look at the point being viewed.
Formula for Height and Distances

Prime Course Trailer

Related Banners

Get PrepInsta Prime & get Access to all 200+ courses offered by PrepInsta in One Subscription

Get Prime

Height and Distance Formulas for Trigonometric ratio

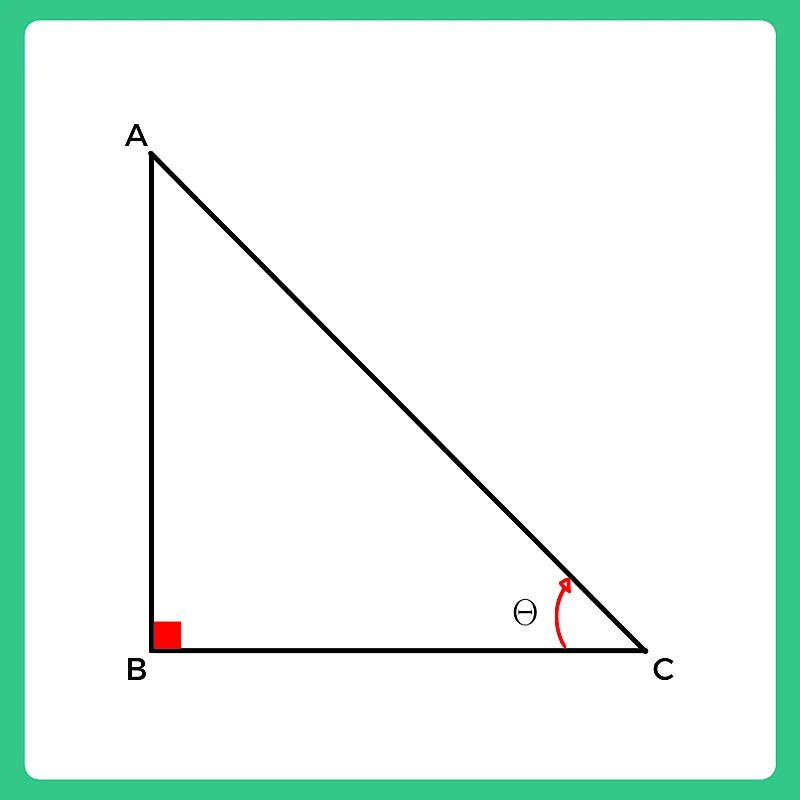
  • ΔABC is a right angled triangle where is AB is the perpendicular, AC is the hypotenuse, and BC is the base.

Then,

  • Sin θ  = \frac{AB}{AC}
  • Cos θ = \frac{BC}{AC}
  • Tan θ  = \frac{AB}{BC}
  • Cosec θ  = \frac{AC}{AB}
  • Sec θ  = \frac{AC}{BC}
  • Cot θ  = \frac{BC}{AB}
  • Trigonometrical Identities:
  • sin2θ + cos2 θ = 1
  • 1 + tan2θ = sec2 θ
  • 1 + cot2θ = cosec2 θ
Trigonometric Formulas for Height and Distances

Definition of Harmonic Progression (H.P)

Harmonic progression is the series when the reciprocal of the terms are in AP. 

For example, \frac{1}{a}, \frac{1}{ (a + d)}, \frac{1}{(a + 2d)}…… are termed as a harmonic progression as a, a + d, a + 2d are in Arithmetic progression. 

  • First term of a HP is \frac{1}{a}
  • There are many Application of Harmonic Progressions.

Some Examples Using Above Formulas:

Question 1:  Varun Observed that from a  point 355 meters away from the foot of a tower, the top of the tower is at an angle of elevation of 30^{o}, then the height (in meters) of the tower is?

Answer: Tan 30° = X/355

= \frac{1}{\sqrt{3}} = X/355

=X= 614.87

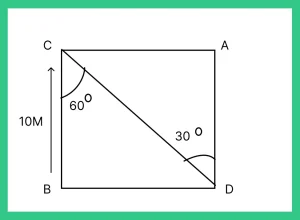
Heights and distance formulas

Question 2: A man is standing in his balcony, which is on the third floor of the building and is at the height of 10m. His angle of elevation at the top of the opposite building is 60^{o} ,and the angle of depression of the base is 30^{o} . Determine the height of the building opposite to him?

Answer: Let AB the building opposite to the man.
Let us assume he is standing at point C
Therefore, we can say that  ∠BCD = 600
∠ACD = 300
Let BD = h
In the given  ADC, tan 300 = 10/CD

  • 1/√3= 10/CD
  • CD = 10 √3
    Also, in the given  ΔDBC, we can say that
    Tan 600 = h/ CD
  • √3= h/ 10 √3
  • H= 30 mtrs
    Therefore, the height of the opposite building is
    AB = BD + DA
    AB = 10 + 30
    AB = 40 mtrs
heights and distance formulas

Question 3: A man stands at the corner of a farm, which is square. The angle of elevation of a scarecrow which presents diagonally opposite corner is60^{o} . When he walks backward in the straight line fir 80ft. The angle of elevation of the scarecrow now becomes 30^{o}. Calculate the field’s area.

Answer: Tan 600 = PQ/QR = √3
PQ= √3QR
Tan 300 = 1/√3 = PQ/SQ
|= PQ/ 80+ QR
80+ QR= √3PQ
80+ QR = 3QR
QR = 40ft

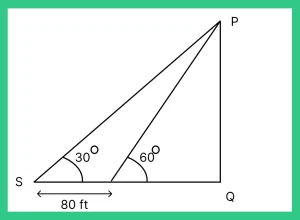
H_D_Question_26

It can also be seen from the figure that the man at point R and the scarecrow at point Q are diagonally opposite to one another.
Therefore, QR is the diagonal of the square farm.
Diagonal =side x  /√2
Therefore, 40 = side x  /√2
Side = 40 √2
Therefore, area = (side)2 = (40 √2)2
= 800 sq feet

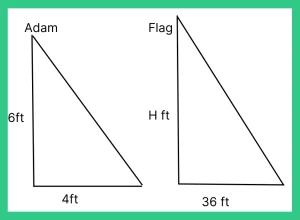
Question 4: Adam is standing in the Sun. He is 6ft tall and is casting a shadow of 4ft. A flag is also present near the man casting a shadow of 36ft. Calculate the height of the flag.
Answer: The angle of elevation for the Sun will be the same for both the cases.
Therefore, the ratio of object to shadow will be the same for both the cases as per the proportionality rule.
Object height/ Shadow height = 6/ 4 = H /36
Therefore, H = 54 ft = Height of the flag

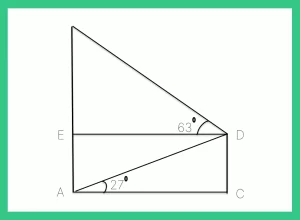
Heights and distance formula

Question 5: When a boy looks from the foot and the top of a tower at the roof of a building, the angles of elevation and depression are 27^{o}and 63^{o}, The height of this building is 40m, then calculate the height of the tower given that tan 630 =2.

Answer: Let the tower be AB
Let the building of height 40m be CD
In the given triangle ACD, AC/DC = cot 270
= cot (90-63)
AC/40 = tan 630 = 2
AC = 80m
Now, DE = AC = 80m
Also, in triangle BED, tan 630 = BE/DE
2= BE/80
Therefore, BE = 160 m
Therefore, the height of the tower can be calculated as AE + EB
= 40 +160 = 200m

heights and distance

Also Check Out

Height and Distance Question and Answers
How to solve Height and Distance questions quickly?
Shortcut and Tricks to solve Height and Distance easily
Branding

Get over 200+ course One Subscription

Courses like AI/ML, Cloud Computing, Ethical Hacking, C, C++, Java, Python, DSA (All Languages), Competitive Coding (All Languages), TCS, Infosys, Wipro, Amazon, DBMS, SQL and others

Get Prime
Course List
Course List

Checkout list of all the video courses in PrepInsta Prime Subscription

Checkout list of all the video courses in PrepInsta Prime Subscription

Checkout
Checkout