Quants Menu
- HCF and LCM
- Number System
- Number Decimals & Fractions
- Surds and Indices
- Divisibility
- Ages
- LCM
- HCF
- Inverse
- Speed Time and Distance
- Work and Time
- Boats and Streams
- Pipes and Cisterns
- Averages
- Allegations and Mixtures
- Ratio and Proportions
- Simple & Compound Interest
- Simple Interest
- Compound Interest
- Percentages
- Profit & Loss
- Successive Discount 1
- Successive Discount 2
- AP GP HP
- Arithmetic Progressions
- Geometric Progressions
- Harmonic Progressions
- Probability
- Permutation & Combination
- Combination
- Circular Permutation
- Geometry
- Heights and Distances
- Perimeter Area and Volume
- Coordinate Geometry
- Venn Diagrams
- Set Theory
- Algebra
- Linear Equations
- Quadratic Equations
- Logarithms
- Clocks
- Calendars
- Clocks and Calendars
- Finding remainder of large powers
PREPINSTA PRIME
Geometry Formulas
Geometry Basic Formulas – Area, Volume and Perimeter
On this page we will discuss about the formulas that are Required to solve Geometry Questions. With Certain Examples which will clear your concept about the topic very well
Basic Geometry Formulas-Area, Volume and Perimeter.
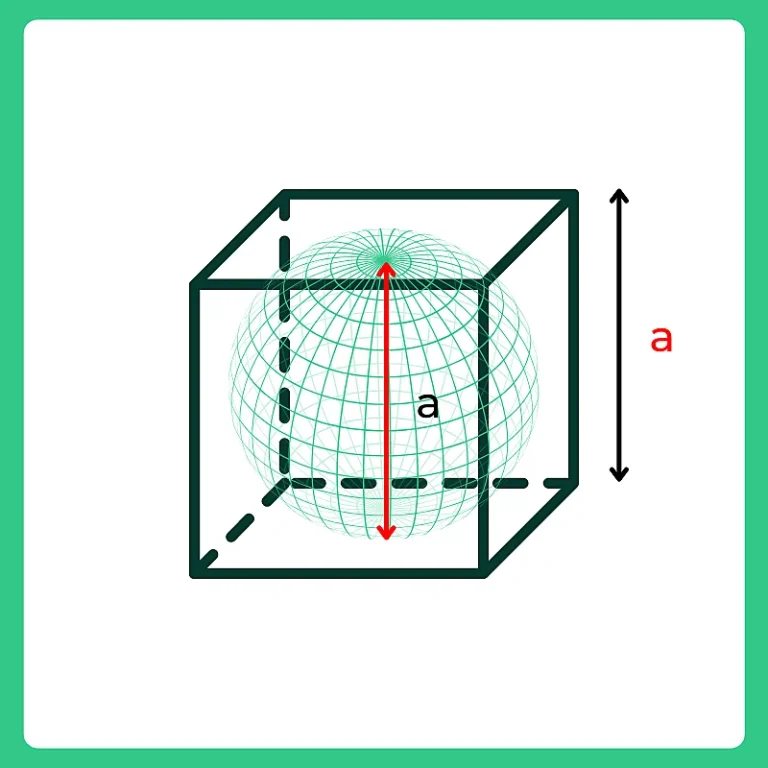
Largest Possible Sphere :
In this type you will be given a cube of “a” cm and will be asked the volume of largest possible sphere which can be chiseled out from it.
Diagonal of the Sphere: \frac{a}{2} = \text{ Radius }
Remaining Empty Space of the Cube: a^3 = \frac{\pi a^3}{6}
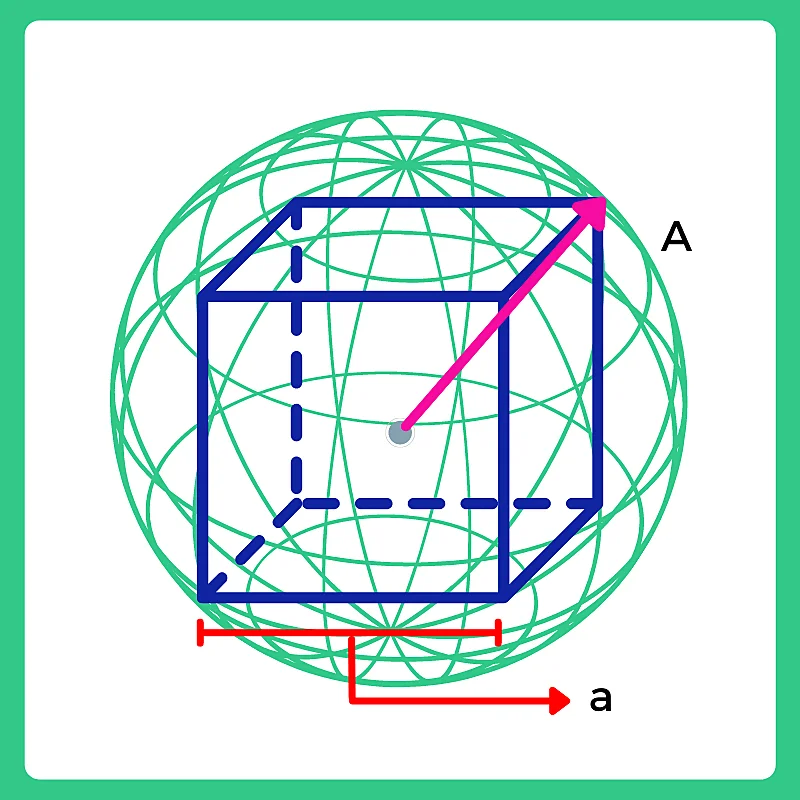
Largest possible cube:
In this type you will be given a sphere of radius “A” cm and will be asked the volume of largest cube which can be chiseled out from it.
Here OA= radius of the sphere. So, diameter of the sphere = 2a
Diagonal of the cube = √3x
(If side of the Square is x)
\Rightarrow \sqrt{3x} = 2a = \Rightarrow x = \frac{2a}{\sqrt3}
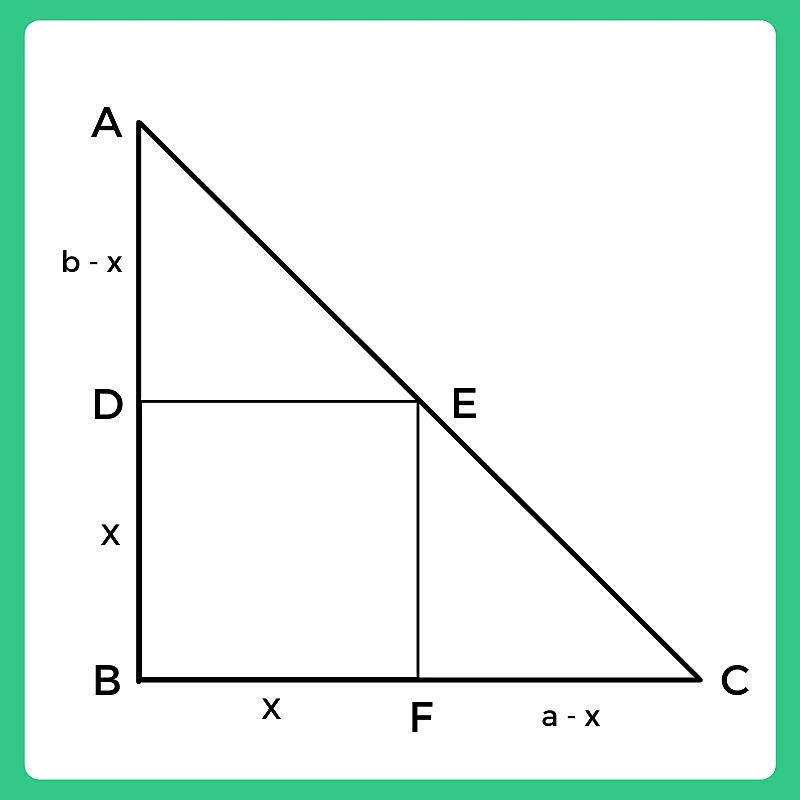
The largest square that can be inscribed in a right angled triangle:
In this type you will be given a Square BDEF when one of its vertices coincide with the vertex of the right angle of the triangle ABC
Side of the Square: \left ( \frac{ab}{a+b}\right )
Area of the Square: \left ( \frac{ab}{a+b}\right )^2
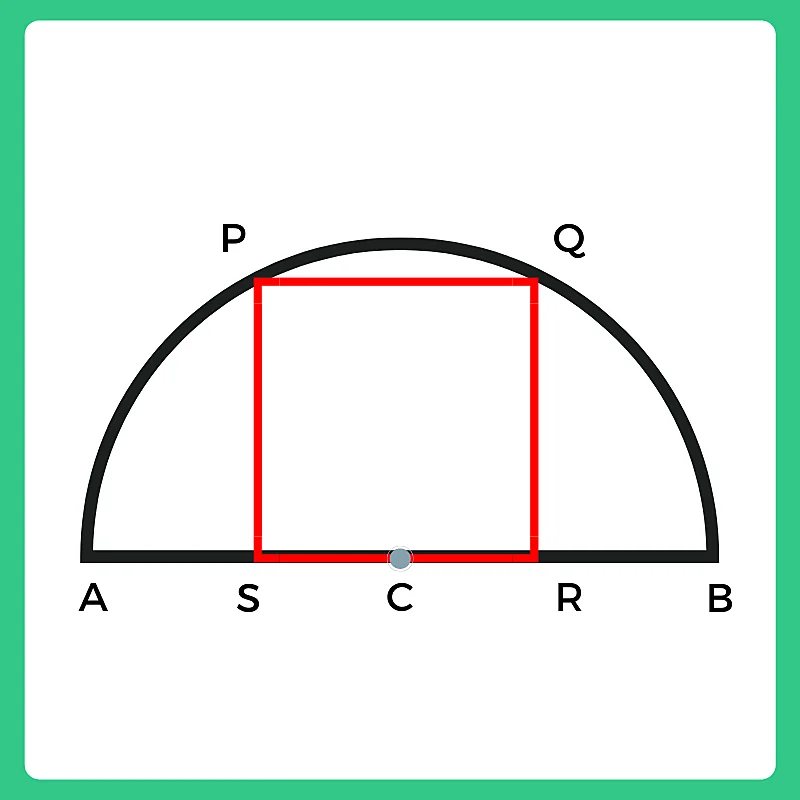
The largest square that can be inscribed in a semi circle:
In this type you will be asked the area of the largest Square which can be inscribed in a Semicircle of radius “r”
Area of the Square: \frac{3}{5}r^2
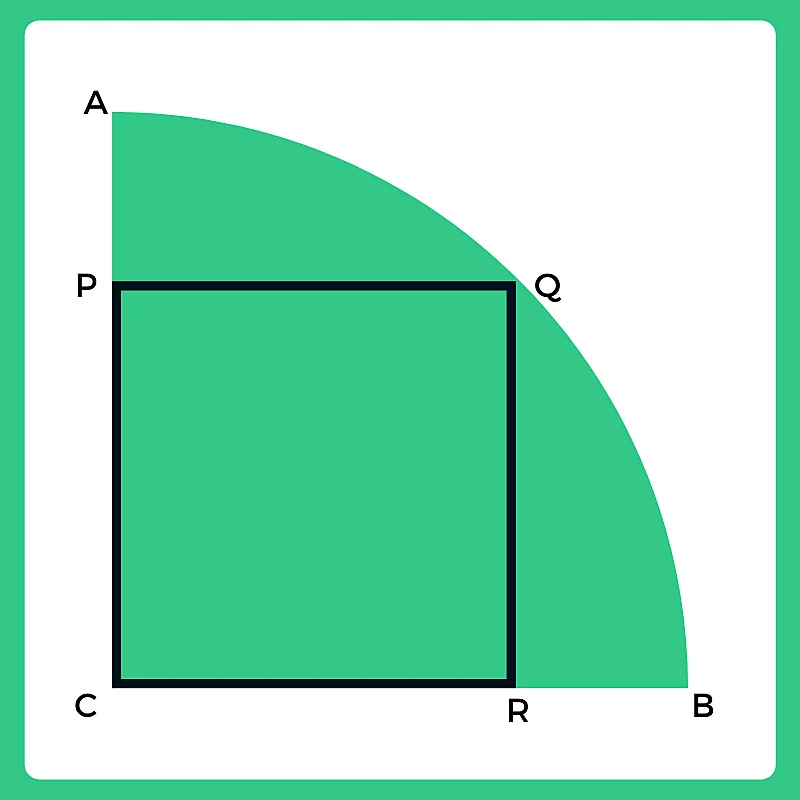
The largest square that can be inscribed in a Quadrant:
In this type you can be asked the area or Side of the largest Square which can be inscribed in a Quadrant of Radius “r”
Side of the Square: \frac{r}{\sqrt2}
Area of Square: \frac{r^2}{2}
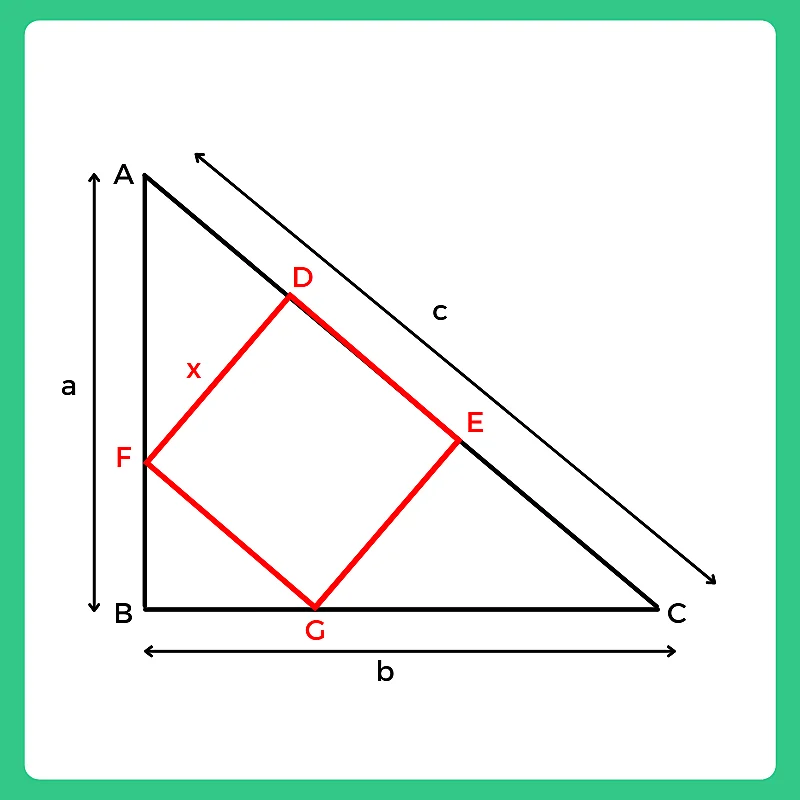
The largest square that can be inscribed in a right angled triangle:
In this type you will be given a Square DEGF when one of its vertices coincide with the hypotenuse of the right angle of the triangle ABC and you can be asked for the side of the square.
Side of the Square: \frac{abc}{a^2 + b^2 + ab}
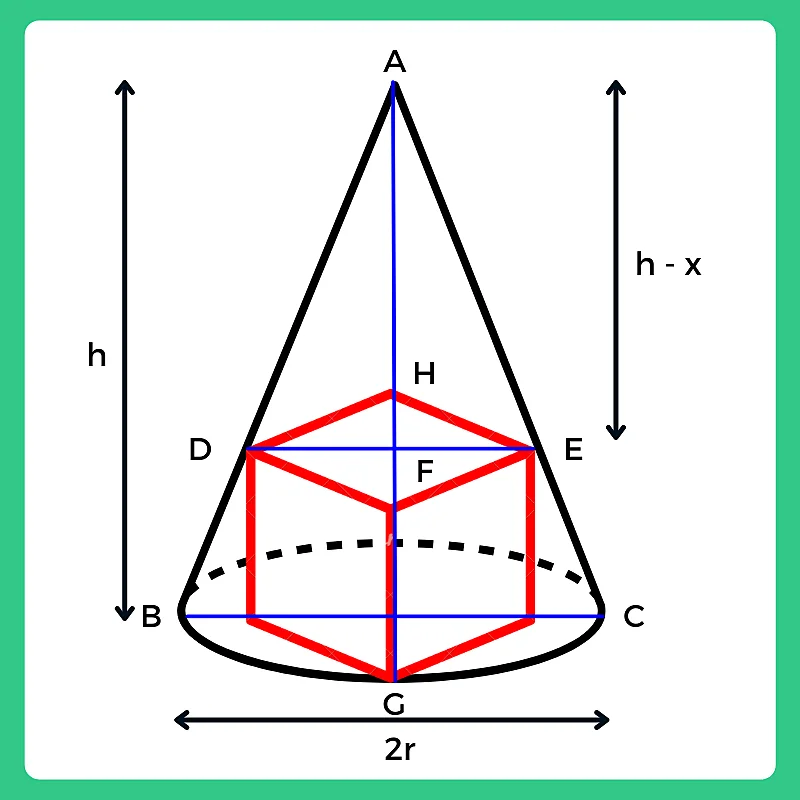
The largest cube that can be chiseled out from a cone:
In this question you will be asked about the side of the largest cube which will be chiseled out of a cone of height ‘h’ cm and radius ‘r’ cm
Side of Cube: \frac{\sqrt2rh}{h + \sqrt2r}
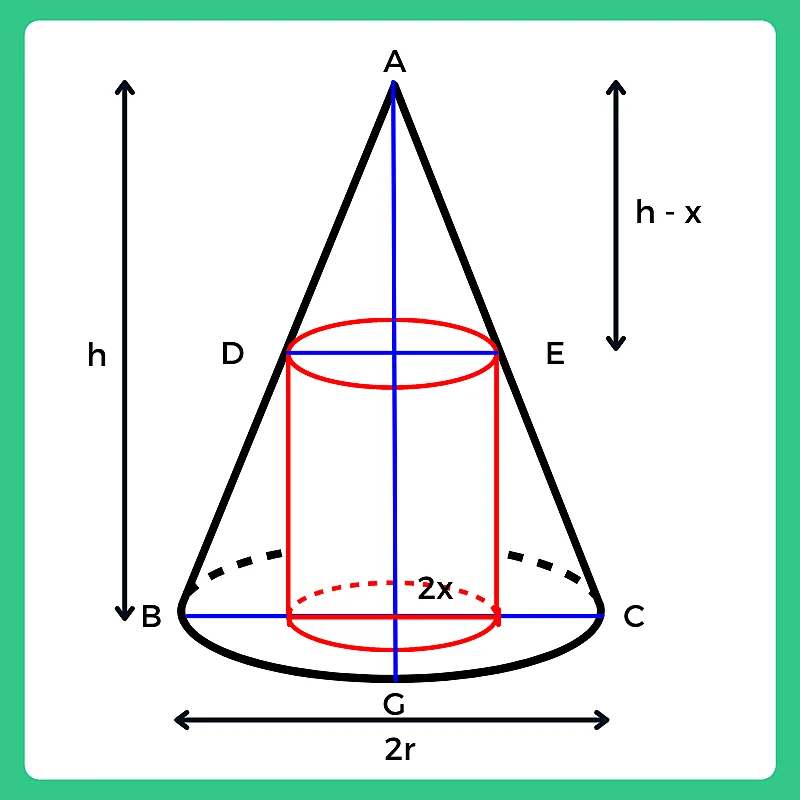
The maximum volume of cylinder:
In this you will be asked about the volume of the largest cylinder which can be chiseled out from a right circular cone.
Maximum Volume of the Cylinder: \pi \times \frac{2r^2}{3}\times \frac{h}{3}
Prime Course Trailer
Related Banners
Get PrepInsta Prime & get Access to all 200+ courses offered by PrepInsta in One Subscription
Some Examples Using above Formulas:
Question 1: Ratan had a hard wooden board out of which he made an equilateral triangle with a side length of 10 cm. Find it’s area?
Answer: The formula to find the area of an equilateral triangle is given by A =\frac{\sqrt{3}}{4}{a}^2
=\frac{\sqrt{3}}{4}{10}^2
=43.30cm^{2}
Question 2: Raj bought a mirror for his room with a length of 12 meters and a diagonal of 15 meters, find the width of the rectangle.
Answer: The width of the rectangle is 9 meters.
In a rectangle, the diagonal divides the rectangle into two congruent right-angled triangles. We can use the Pythagorean Theorem to find the width (‘b’) of the rectangle using the length (‘a’) and the diagonal (‘c’):
b^{2} =c^{2} – a^{2}
b^{2} = 15^{2} – 12^{2}
b^{2}=225-144
b^{2}= 81
b=\sqrt{81}
b = 9 m
Question 3: Sam made a circle out of clay find it’s area in terms of pi given the diameter of circle is 18cm?
Answer: Given Diameter = 18 cm
Radius = 9 cm
Area of circle = \pi r^{2}
Area = \pi 9^{2}
Area= 81\picm^{2}
Question 4: Find Volume of a ice-cream cone whose radius is 6 cm and height 7 cm?
Answer: We know Volume of cone = \frac{1}{3}\pi r^{2}h
Hence, \frac{1}{3}\pi r^{2}h = \frac{1}{3}\pi 6^{2}7
=84\picm^{3}
Question 5: The supplement of 90^{\cdot } is?
Answer: Supplementary angles means those angles whose sum is 180.
According to question:
90^{\cdot } + x = 180
= 180- 90 = x
= 90^{\cdot }
Also Check Out
Get over 200+ course One Subscription
Courses like AI/ML, Cloud Computing, Ethical Hacking, C, C++, Java, Python, DSA (All Languages), Competitive Coding (All Languages), TCS, Infosys, Wipro, Amazon, DBMS, SQL and others
- Heights and Distances – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Perimeter Area and Volume – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Coordinate Geometry – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Venn Diagrams – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Set Theory – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Heights and Distances – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Perimeter Area and Volume – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Coordinate Geometry – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Venn Diagrams – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Set Theory – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts

 Apply For Jobs
Apply For Jobs Get Hiring Updates
Get Hiring Updates




Login/Signup to comment