Logical Menu
- Number Series
- Coding and Number Series
- Letter and Symbol Series
- Logical Sequence of Words
- Analogy and Classification Pattern
- Statements and Conclusions
- Statements and Assumptions
- Data Sufficiency
- Visual Reasoning
- Cube and Cuboid
- Cube
- Dice
- Directional Senses
- Blood Relations
- Odd Man Out
- Syllogism
- Arrangements
- Seating Arrangements
- Coding Deductive Logic
- Objective Reasoning
- Selection Decision Tables
- Attention to Details
- Inferred Meaning
- Cryprtarithmetic
- Get Off-campus Drive Updates
- Get Hiring Updates
- Contact US
PREPINSTA PRIME
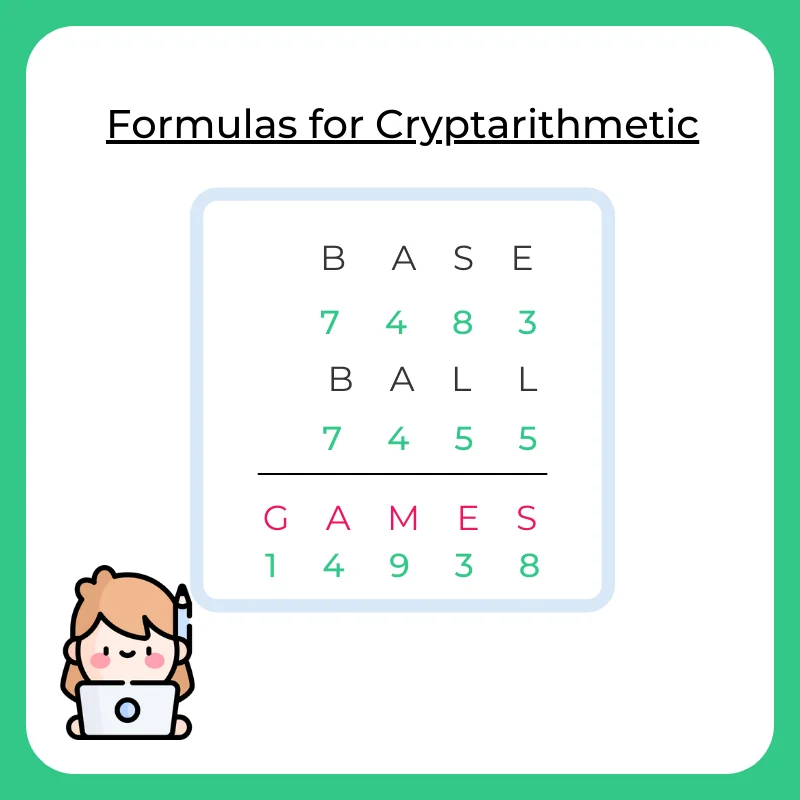
Formulas For Cryptarithmetic
Cryptarithmetic Formulas
Go through the page to learn the important Formulas for Cryptarithmetic which will help you to solve the related problem asked in Companies Hiring Test and other Competitive Exams for getting Jobs.
Types of Cryptarithmetics
Cryptarithmetic is considered to be, both a science as well as an art. Hence apart from logic, one must use his/her presence of mind and a little bit of common sense to solve the problems.
Usually, all the Cryptarithmetic questions follow the same pattern, which, makes it clear that there are no different types of questions asked on Cryptarithmetic.
However, certain tips and tricks have to be followed to solve the questions quickly and easily. All of which are mentioned in the continuing tips and tricks page.
Types of cryptarithm include the alphametic, the digimetic, and the skeletal division.
Type 1. Alphametic
- A type of cryptarithm in which a set of words is written down in the form of a long addition sum or some other mathematical problem. The object is to replace the letters of the alphabet with decimal digits to make a valid arithmetic sum.
Type 2. Digimetic
- A cryptarithm in which digits are used to represent other digits.
Type 3. Skeletal division
- A long division in which most or all of the digits are replaced by symbols (usually asterisks) to form a cryptarithm.
Type 4. Reverse cryptarithm
- A rare variation where a formula is written and the solution is the corresponding cryptarithm whose solution is the formula given.
Prime Course Trailer
Related Banners
Get PrepInsta Prime & get Access to all 200+ courses offered by PrepInsta in One Subscription
Also Checkout
Sample Cryptarithmetic Questions with Solution
Question: 1
WORLD+TRADE=CENTER value of C+E+N+T+E+R
Options:
a. 45
b. 25
c. 27
d. 36
Answer: c. 27
Explanation:
WORLD + TRADE=CENTER
W O R L D
T R A D E
——————
C E N T E R
So,start from last C the value is 1 always.
Next W+T=E right.
By adding numbers we should get 1 as a carry (which is C value).
So,take W as 5 and T as 7 and add now which is 12.
NO TWO ALPHABETS SHOULD HAVE SAME VALUES ALWAYS.
Do it accordingly.
5 3 6 8 4
7 6 0 4 2
—————-
1 2 9 7 2 6
53684+ 76042=129726
C+E+N+T+E+R = 27
Question: 2
MAC + MAAR = JOCKO, find the value of 3A + 2M + 2C
Options:
a. 32
b. 33
c. 31
d. 39
Answer: c. 31
Explanation:
M A C
+ M A A R
——————
J O C K O
Step 1:
Here obviously J = 1 as max carry allowed it
(nothing) + M = O, i.e. 0 + M = O this should have had been M only
It means that there is 1 carry coming from previous step
Also this M + 1 (carry) = O, is generating 1 carry to next step
This means M + 1 > 10, this is only possible when M = 9
=> O = M + 1 = 9 + 0 = 0 (1 carry)
9 A C + 9 A A R ———– 1 0 C K 0
Step 2:
C + R = 0
Since, this is at units place there can be no carry from previous step
Possible values of (C, R) can be (2, 8), (3,7), (4, 6), (6,4), (7,3), (2,8)
Let’s assume, first case C = 2 and R = 8 in that case C + R, will generate 1 carry to next step A + A = K.
New problem looks like this –
9 A 2
+ 9 A A 8
—————-
1 0 2 K 0
Step 3:
Thus, A + A + 1 (Carry) = K
Also, 9 + A = 2, this is possible in two ways
If, A = 3 (no carry from previous step) 9 + 3 = 2 (1 carry to next step)
If, A = 2 (1 carry from the previous step) but C value is already assumed to be 2, so this is not possible thus, A has to be 3
Thus K = 3 + 3 + 1 = 7
All values are fetched and there is no conflict.
9 3 2
+ 9 3 3 8
—————
1 0 2 7 0
Step 4:
Thus, 3 A + 2 M + 2 C = 3(3) + 2(9) + 2(2)
= 9 + 18 + 4 = 31
Question: 3
NO + GUN + NO = HUNT, Find the value of HUNT.
Options:
a. 1079
b. 1080
c. 1088
d. 1082
Answer: d. 1082
Explanation:
N O
+ G U N
N O
—————
H U N T
Step 1: Here H = 1, from the NUNN column we must have “carry 1”, so G = 9, U = zero.
Since we have “carry” zero or 1 or 2 from the ONOT column,
Correspondingly we have N + U = 10 or 9 or 8. But duplication is not allowed, so N = 8 with “carry 2” from ONOT.
Hence, O + O = T + 20 – 8 = T + 12.
Testing for T = 2, 4 or 6, we find only T = 2 acceptable, O = 7.
So we have 87 + 908 + 87 = 1082
Question: 4
P A R I S + F R A N C E = L O N D O N. Find the value of LONDON.
Options:
a. 23
b. 25
c. 27
d. 29
Answers: a.23
Explanation:
Step 1:
Starting from the rightmost column, we have S + E = N.
Since no two letters can have the same value, S and E must be different digits.
The pairs of digits that sum to a number ending in N (N can be 0 or 1, considering the carry from the next column) are (S=7, E=9) or (S=8, E=1). Let’s consider the first pair: S=7 and E=9.
This means N = 6 (from the carry).
Step 2:
In the next column, we have I + C + N (carry from the previous step) = O. Since I + C must be at least 10 (assuming I=1 and C=9), O = 0.
Step 3:
Now, we have R + E + N (carry from the previous step) = N. Since N is 6 (from Step 1), the only valid pair is (R=2).
Step 4:
In the following column, A + F + N (carry from the previous step) = D. Since D can’t be 0 (from Step 2), the only valid option is (A=5 and F=8).
Step 5:
In the leftmost column, P + R + L (carry from the previous step) = L. Since no two letters can have the same value, P and R must be different.
The pairs of digits that sum to a number ending in L (L can be 0 or 1, considering the carry from the next column) are (P=4, R=6) or (P=3, R=7).
Let’s consider the first pair: P=4 and R=6.
This means L = 1 (from the carry).
Now we can calculate the sum:
P + A + R + I + S = 4 + 5 + 6 + 1 + 7 = 23
So, P + A + R + I + S = 23.
Therefore, the correct answer is 23.
Question: 5
S T A R S + P L U T O = P L A N E T. Find the value of PLANET.
Options:
a. 23
b. 21
c. 27
d. 29
Answer: b. 21
Explanation:
Step 1:
Starting from the rightmost column, we have S + O = T.
Since no two letters can have the same value, S and O must be different digits.
Pairs of digits that sum to a number ending in T (T can be 0 or 1, considering the carry from the next column) are (S=7, O=9) or (S=8, O=1).
Let’s consider the first pair: S=7 and O=9. This means T = 6 (from the carry).
Step 2:
In the next column, we have R + U + N (carry from the previous step) = E.
Since E can’t be 0 (from the leftmost column), the only valid option is (R=3, U=2, and N=1).
Step 3:
Now, we have A + T + N (carry from the previous step) = T.
Since T is 6 (from Step 1) and A + N must be at least 10, the only valid pair is (A=4 and N=7).
Step 4:
In the leftmost column, S + P (carry from the previous step) = P.
Since no two letters can have the same value, S and P must be different.
Pairs of digits that sum to a number ending in P (P can be 0 or 1, considering the carry from the next column) are (S=5, P=6) or (S=4, P=5).
Let’s consider the first pair: S=5 and P=6. This means the carry to the next column is 1.
Now we can calculate the sum:
S + A + T + U + R + N = 5 + 4 + 6 + 2 + 3 + 1 = 21
So, S + A + T + U + R + N = 21
Also Checkout
Get over 200+ course One Subscription
Courses like AI/ML, Cloud Computing, Ethical Hacking, C, C++, Java, Python, DSA (All Languages), Competitive Coding (All Languages), TCS, Infosys, Wipro, Amazon, DBMS, SQL and others
- Cryprtarithmetic – Questions Formulas | How to Solve Quickly | Tricks & Shortcuts
- Cryprtarithmetic –
Questions
Formulas |
How to Solve Quickly |
Tricks & Shortcuts

 Apply For Jobs
Apply For Jobs Get Hiring Updates
Get Hiring Updates




Login/Signup to comment