Quants Menu
- HCF and LCM
- Number System
- Number Decimals & Fractions
- Surds and Indices
- Divisibility
- Ages
- LCM
- HCF
- Inverse
- Speed Time and Distance
- Work and Time
- Boats and Streams
- Pipes and Cisterns
- Averages
- Allegations and Mixtures
- Ratio and Proportions
- Simple & Compound Interest
- Simple Interest
- Compound Interest
- Percentages
- Profit & Loss
- Successive Discount 1
- Successive Discount 2
- AP GP HP
- Arithmetic Progressions
- Geometric Progressions
- Harmonic Progressions
- Probability
- Permutation & Combination
- Combination
- Circular Permutation
- Geometry
- Heights and Distances
- Perimeter Area and Volume
- Coordinate Geometry
- Venn Diagrams
- Set Theory
- Algebra
- Linear Equations
- Quadratic Equations
- Logarithms
- Clocks
- Calendars
- Clocks and Calendars
- Finding remainder of large powers
PREPINSTA PRIME
Arithmetic Progression Questions and Answers
Arithmetic Progression Questions and Answers for Practice
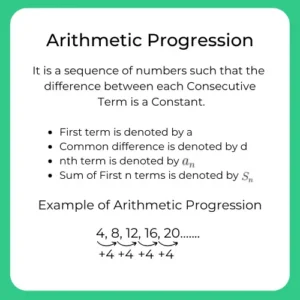
There are a number of Arithmetic progression Questions and they have varied formulas for solving the sequence. In this page Arithmetic Progression Questions and Answers are given.
Notations in Arithmetic Progression (AP)
- Initial term (a): In an arithmetic progression, the first number in the series is called the initial term.
- Common difference (d): The ‘d’ denotes the variance between all the consecutive terms of the progression.
- Nth term (a_{n}): The a_{n} defines the terms of the progression or sequence whereas ‘n denotes the position of the given number or alphabet which can be obtained by a_{n} = a + (n − 1)d.
- Sum of AP (Sn): The sum of an AP can be obtained by Sn = \frac{n}{2} [2a + (n − 1) d]
If ‘n’ is equal to one then it denotes the initial term of arithmetic progression. This is known as the precise method for an Arithmetic progression.
General form of AP:
The general form of an AP is : a, a + d, a + 2d, a + 3d, …….
Finite or Infinite Arithmetic Progressions
- Finite Arithmetic Progression
When there are limited number of terms in the sequence then it is known as Finite Arithmetic Progression.
For example: 10, 20, 30, 40, 50
- Infinite Arithmetic Progression
When there are unlimited number of terms in the sequence then it is known as Infinite Arithmetic Progression.
For example: 3, 5, 7, 9, 11, 13, ..…….
(1) If the difference (d) is positive, the terms of the sequence will increase towards positive infinity.
(2) If the difference is negative, the terms of the sequence will increase towards negative infinity.
Prime Course Trailer
Related Banners
Get PrepInsta Prime & get Access to all 200+ courses offered by PrepInsta in One Subscription
Also Check Out
Arithmetic Progression Questions and Answers

Please login to report
Also Check Out
Get over 200+ course One Subscription
Courses like AI/ML, Cloud Computing, Ethical Hacking, C, C++, Java, Python, DSA (All Languages), Competitive Coding (All Languages), TCS, Infosys, Wipro, Amazon, DBMS, SQL and others
- AP GP HP – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Geometric Progressions – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Harmonic Progressions – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- AP GP HP –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Geometric Progressions –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Harmonic Progressions –
Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts

 Apply For Jobs
Apply For Jobs Get Hiring Updates
Get Hiring Updates




Login/Signup to comment