Quants Menu
- HCF and LCM
- Number System
- Number Decimals & Fractions
- Surds and Indices
- Divisibility
- Ages
- LCM
- HCF
- Inverse
- Speed Time and Distance
- Work and Time
- Boats and Streams
- Pipes and Cisterns
- Averages
- Allegations and Mixtures
- Ratio and Proportions
- Simple & Compound Interest
- Simple Interest
- Compound Interest
- Percentages
- Profit & Loss
- Successive Discount 1
- Successive Discount 2
- AP GP HP
- Arithmetic Progressions
- Geometric Progressions
- Harmonic Progressions
- Probability
- Permutation & Combination
- Combination
- Circular Permutation
- Geometry
- Heights and Distances
- Perimeter Area and Volume
- Coordinate Geometry
- Venn Diagrams
- Set Theory
- Algebra
- Linear Equations
- Quadratic Equations
- Logarithms
- Clocks
- Calendars
- Clocks and Calendars
- Finding remainder of large powers
PREPINSTA PRIME
Alligations and Mixtures Questions and Answers
Alligations and Mixtures Questions
Alligation and Mixtures Questions and Answers are provided on this page for students to practice and get an idea how this topic is asked in the exam.
Alligation is the process which allows us to identify the proportion in which the numerous ingredients must be mixed at the specified price in order to create a mixture at a given price.
Alligation Methods
Alligation Medial which is used to calculate the quantity of a mixture.
Alligation Alternate which is used to calculate the quantity of each ingredient required to produce a mixture.

Alligation and Mixture Rules
- If two different ingredients are assorted in a proportion, then
- \frac{( \text{ Quantity of cheaper})}{ (\text{ Quantity of dearer})}= \frac{(C.P. of dearer)-(Mean Price)}{ (Mean Price)-(C.P.of Cheaper)}
- Furthermore, it can be presented as:
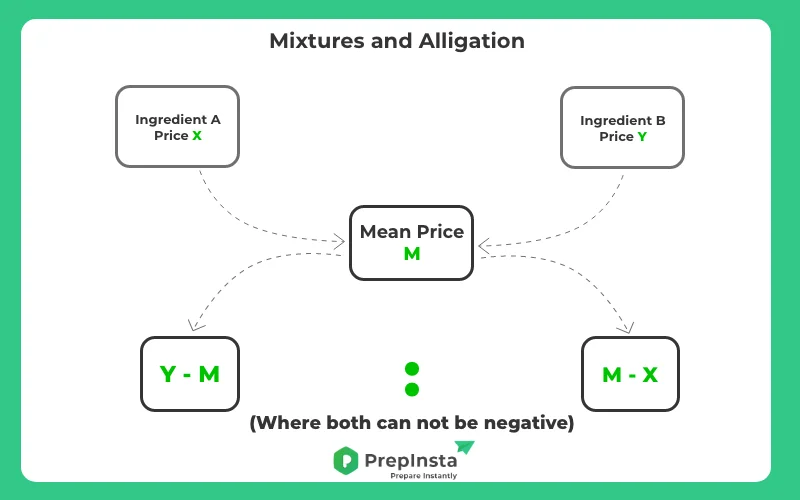
- (Cheaper quantity): (dearer quantity) = (d – m): (m – c)
- Repeated Dilution: If a barrel contains ‘a’ units of liquid out of which ‘b’ units are removed and substituted by water. Then, after ‘n’ processes, the amount of liquid,
= [x (1 – y/x)^{n}] units - Furthermore, it is used to analyze the quantity of pure liquid left after ‘n’ number of procedures of constant substitution is prepared on the pure quantity.
Prime Course Trailer
Related Banners
Get PrepInsta Prime & get Access to all 200+ courses offered by PrepInsta in One Subscription
Also Check Out
Practice Alligation and Mixtures Questions

×
Please login to report
Also Check Out
Get over 200+ course One Subscription
Courses like AI/ML, Cloud Computing, Ethical Hacking, C, C++, Java, Python, DSA (All Languages), Competitive Coding (All Languages), TCS, Infosys, Wipro, Amazon, DBMS, SQL and others
- Averages – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Ratio and Proportions – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Simple & Compound Interest – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Simple Interest – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Compound Interest – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Percentages – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
- Profit & Loss – Questions | Formulas | How to Solve Quickly | Tricks & Shortcuts
Others
- Averages – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Ratio and Proportions – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Simple & Compound Interest – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Simple Interest – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Compound Interest – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Percentages – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts - Profit & Loss – Questions |
Formulas |
How to Solve Quickly |
Tricks & Shortcuts
Others

 Apply For Jobs
Apply For Jobs Get Hiring Updates
Get Hiring Updates




Login/Signup to comment