Once you attempt the question then PrepInsta explanation will be displayed.
First we fix 1 at unit place of a six digit number.
Since any number can be divided by 1 .
So it does not matter what comes at other five places .
_ _ _ _ _ 1
So, first position can be filled in 5 ways as any number
from 2 to 6 can be placed in that position .
Similarly 2nd position can be filled with 4 ways .
So , by fundamental principle of counting ,
We get,
5x4x3x2x1x1=120 or 5! x 1=120
Now for 2 at units place ,
Any even number is divided by 2 ,
Hence it is again similar to first case,
5! × 1 =120
Now for 3 at units place ,
For any number to be divisible by 3 , we need to make
sure that sum of the digits is divisible by 3.
Now , 1+ 2 + 3 +4 + 5 +6 = 21
Since 21 is divisible by 3 , so any number formed by number
1 to 6 is divisible by 3 .
Hence , 5! × 1 =120.
Similarly for 5 , 5! ×1 = 120
Now for 6 at unit place ,
We know that any number which is divisible by 2 and
3 is also divisible by 6 .
Hence , 5! ×1 =120
Now for 4 at unit place.
If there is even number at tens place with 4 at units place ,
then the number is divided by 4 else no.
So we have only two even number 2 and 6 which we can
put at tens place .
So now first fixing 2 at tens place and 4 at units place .
_ _ _ _ 2 4
We get by fundamental principle of counting ,
4 × 3 × 2 × 1 × 1 ×1
Or
4! × 1 ×1 = 24
Similarly for 6 at tens place and 4 at units place .
We get 4! × 1 × 1 = 24
Now let us add all the values we got ,
120 +120 +120 + 120 +120 +24 +24 = 648
Hence answer is (c)


 Apply For Jobs
Apply For Jobs Get Hiring Updates
Get Hiring Updates











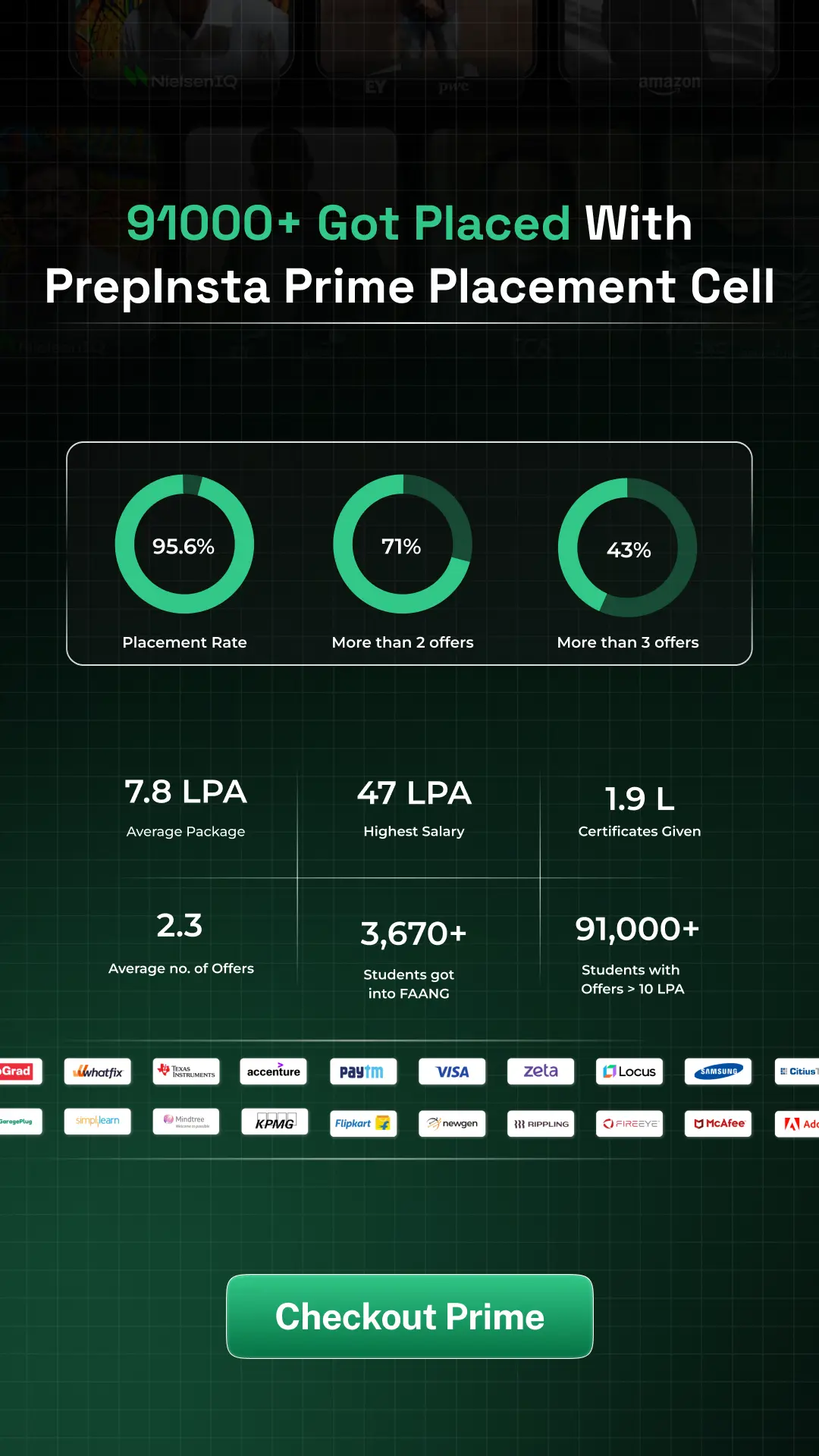







it really good test.
Perfectly worth it ..