6 Digit Number Puzzle
6 Digit Number Puzzle
How many six-digit numbers can be formed using the digits 1 to 6 exactly once, such that the number is divisible by the digit in its unit place (i.e., the last digit)?
In other words, from all possible 6-digit numbers with no repeated digits from 1 to 6, how many are divisible by their last digit?

Solution: 6 Digit Number
Divisibility Rule Analysis
Divisibility Rule of 1

Divisibility Rule of 2

Divisibility Rule of 3

Divisibility Rule of 4

Divisibility Rule of 5

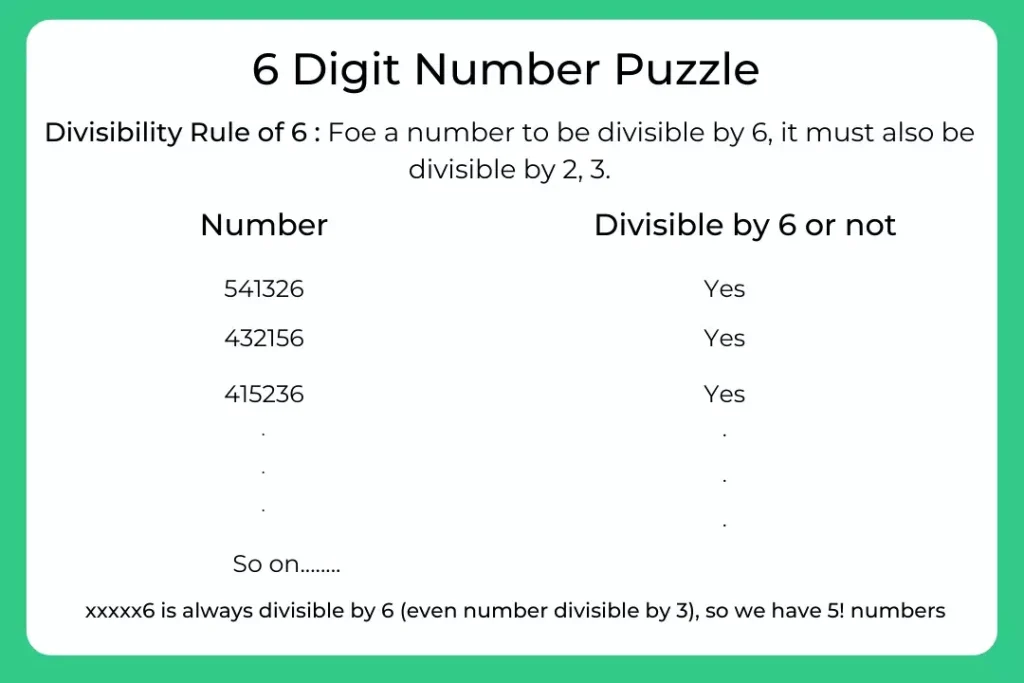
Divisibility Rule of 6
Detailed Analysis
Explanation-
XXXXX1 is always divisible by 1, so we have 5! numbers.
XXXXX2 is always divisible by 2, so we have 5! numbers.
XXXXX3 is always divisible by 3 (sum of digits is always 21), so we have 5! numbers.
XXXXY4 is divisible by 4 only if Y is 2 or 6, so we have 2 \times 4! numbers.
XXXXX5 is always divisible by 5, so we have 5! numbers.
XXXXX6 is always divisible by 6 (even number divisible by 3), so we have 5! numbers.
So total number of numbers with required property
= 5 \times 5! + 2\times 4! = 600 + 48 = 648 numbers.

Also Check:
- Flipping Coins Puzzle Answer
- Bag of Forgery Coins Puzzle Answer
- 3 Glass & 10 Coins Puzzle Answer
- Pirates and 100 Coins Puzzle Answer
- Horse Puzzle Answer
- Handshake Puzzle Answer
- Burning Rope Puzzle Answer
- Shopkeeper & the fake note Puzzle Answer
- Heavier Ball Puzzle Answer
- 5L measuring Puzzle Answer
- Gold Bar Puzzle Answer




Login/Signup to comment