Construct Tree from given Postorder and Inorder Traversals

March 25, 2025

Construct Tree From Given Inorder and Postorder Traversals in Java

There are three types of traversals in a tree: Inorder, Preorder and Postorder traversal. A tree can be formed with any two tree traversals in which one of them being the in order traversal.

construct a tree from preorder and inorder traversal

Construct Tree From Given Inorder and Postorder Traversals

Example

Given traversals:
Postorder: 12, 30, 40, 37, 25, 60, 70, 62, 87, 75, 50
Inorder:12, 25, 30, 37, 40, 50, 60, 62, 70, 75, 87
1. The last element in the postorder traversal is the root of the tree.
So, here 50 will be the root of the tree.
2. We will find the index of 50 in the inorder traversal.The index found is 5. Let this index is denoted by ‘pos’.
3. All the elements to the left of this index ( from 0 to 4 index) will be in the left subtree of 50.
4. And all the elements to the right of this index ( from 6 to 10) will be in the right subtree of 50.

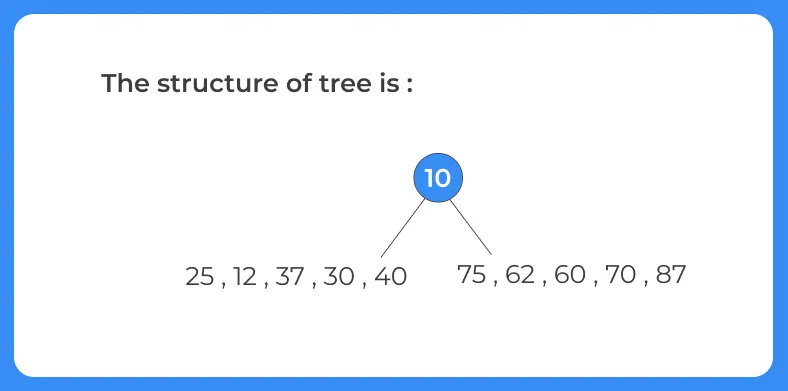
structure of tree

Find size of the Binary tree

Find the height of binary tree

Find maximum in binary tree

Check whether two tree are identical

Spiral Order traversal of Tree

Now, we will divide preorder and inorder array in two parts.
One is for the left subtree and other is for the right subtree.

Let

  • psi: starting index for preorder array
  • pei: ending index for preorder array
  • isi: starting index of inorder array
  • iei: ending index of preorder array
  • clc: Number of elements in the left subtree

Clearly, clc= pos – isi;

For left subtree:
Postorder array: from index psi, psi + clc – 1
Inorder array: from index isi, isi + clc -1

For right subtree:
Postorder array: from index psi+clc, pei – 1
Inorder array: from index isi + clc + 1, iei


Using the above arrays, all the steps are recursively repeated.
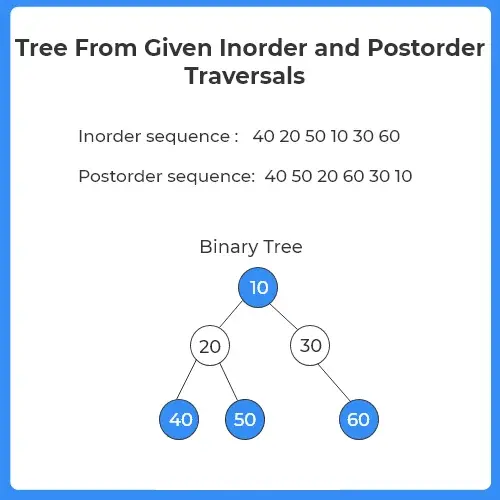
The following binary tree is obtained:

Construct Tree from given Inorder and Postorder traversals

Code For Binary Search Tree​

Run 
public class Main
{

// Binary tree class
  public static class BinaryTree
  {
// Node class
    public class Node
    {
      int data;
      Node left;
      Node right;

      public Node (int data)
      {
	this.data = data;
	this.left = null;
	this.right = null;
      }

    }

    private Node root;
    private int size;

    public BinaryTree (int[]post, int[]in)
    {
      this.root =
	this.construct (post, 0, post.length -1, in, 0, in.length-1);

    }

    private Node construct (int[]post, int psi, int pei, int[]in, int isi,
			    int iei)
    {

// Base case
      if (psi > pei)
	{
	  return null;
	}

      Node node = new Node (post[pei]);
      node.left = null;
      node.right = null;
      this.size++;

// Searching post[pei] in inorder array
      int pos = -1;
      for (int i = isi; i <= iei; i++)
	{
	  if (in[i] == node.data)
	    {
	      pos = i;
	      break;
	    }
	}

// Number of elements in left subtree
      int clc = pos-isi;

// Left subtree
      node.left =
	this.construct (post, psi, psi + clc-1, in, isi, isi + clc-1);

// Right subtree
      node.right =
	this.construct (post, psi + clc, pei-1, in, isi + clc + 1, iei);

      return node;
    }

// Postorder tree traversal
    public void postOrder ()
    {
      postOrder (this.root);
    }

    private void postOrder (Node node)
    {
      if (node == null)
	{
	  return;
	}

      postOrder (node.left);
      postOrder (node.right);
      System.out.print (node.data + " ");
    }
  }

  public static void main (String[]args) throws Exception
  {

// Construct binary tree

    int[] in = { 12, 25, 30, 37, 40, 50, 60, 62, 70, 75, 87 };
    int[] post = { 12, 30, 40, 37, 25, 60, 70, 62, 87, 75, 50 };

    BinaryTree bt = new BinaryTree (post, in);
    System.out.println("The new tree constructed is (Postorder) :  ");
    bt.postOrder ();

  }
}

Output :

The new tree constructed is (Postorder) :  
12 30 40 37 25 60 70 62 87 75 50